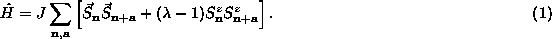
Consider the classical 2D-model of a Heisenberg easy-plane AFM, with the Hamiltonian
Here J > 0 is the exchange constant,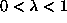 
describes
anisotropy with the xy-plane as the easy-plane.
Spins 
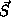are classical vectors on a 2D-square lattice with lattice
constant a.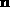 
denotes lattice sites of one sublattice,
and 
's are the set of displacements to the
nearest-neighbors on the other sublattice. We are interested in
the small anisotropy case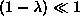 
.
A continuum model of AFM's can be derived from (1) in
the usual way, see [2],[4],[6]. We
define the magnetization vector 
and the sublattice
magnetization vector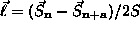 
on the set of nearest-neighbor
pairs, with the constraints 
, 
.
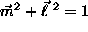Then, for low frequencies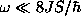 
and small gradients,
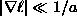, the magnetization of an AFM is
small, 
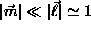. The
magnetization can be considered as a ``slave'' variable, and can
be expressed as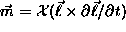 
, where 
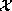is a
susceptibility defined below [Eq. (3)]. After
eliminating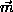 
one obtains equations for 
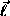only.
Using the usual angular variables, (
) these equations can be
written in the form[4],[6]
where c is the magnon phase velocity,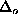 
is
the characteristic length scale. For the Hamiltonian
(1)
