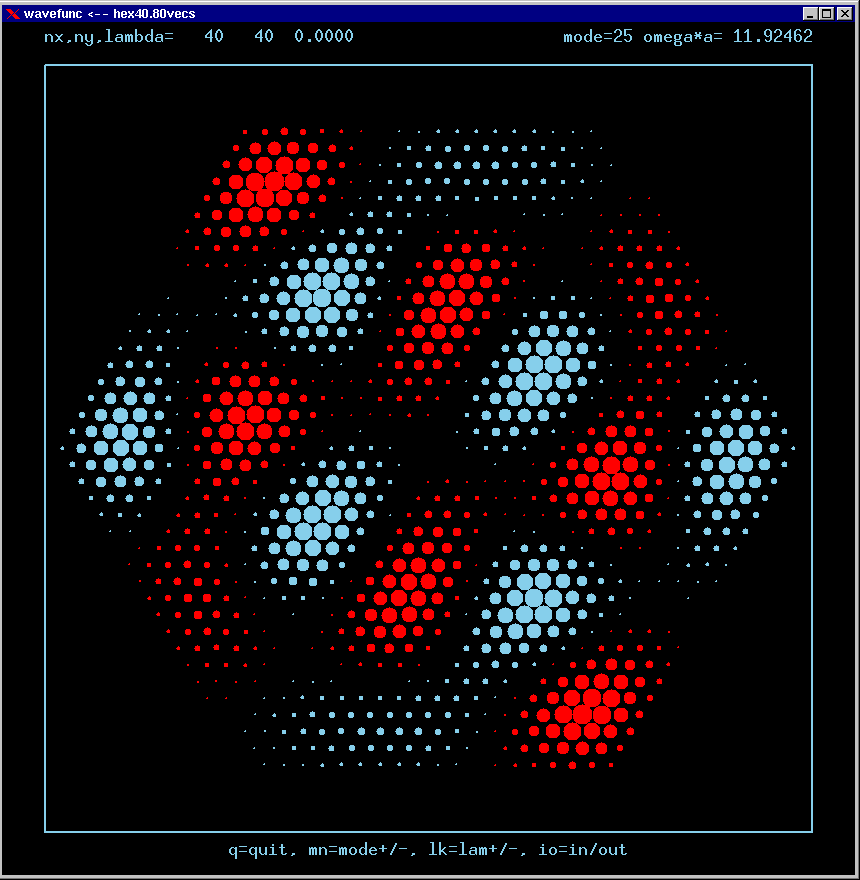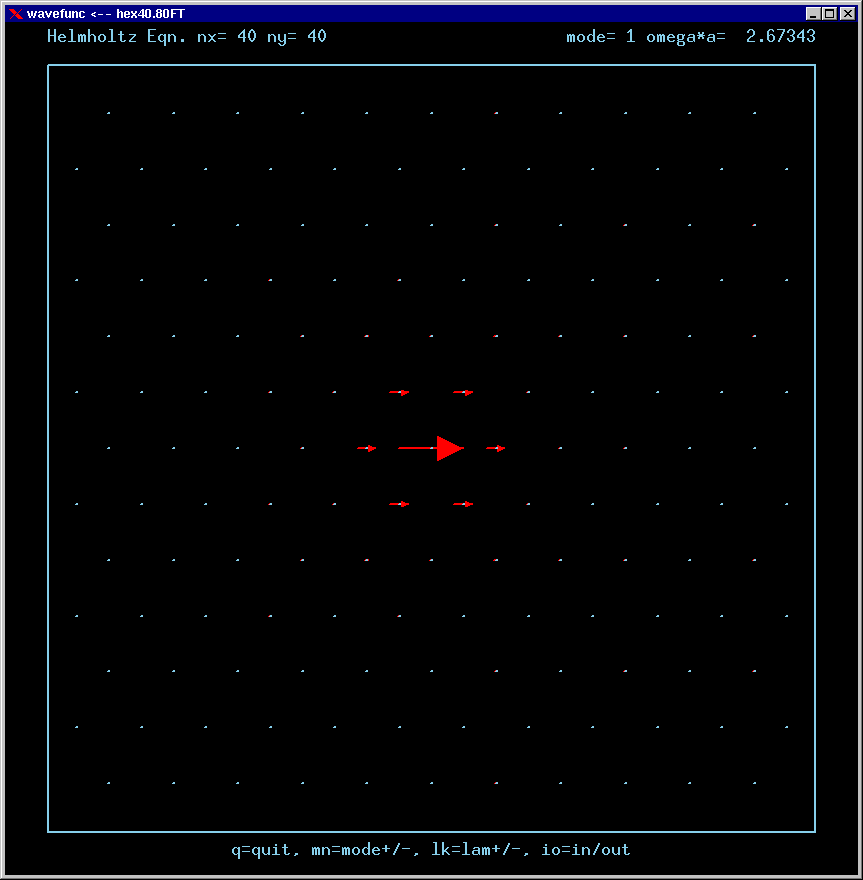
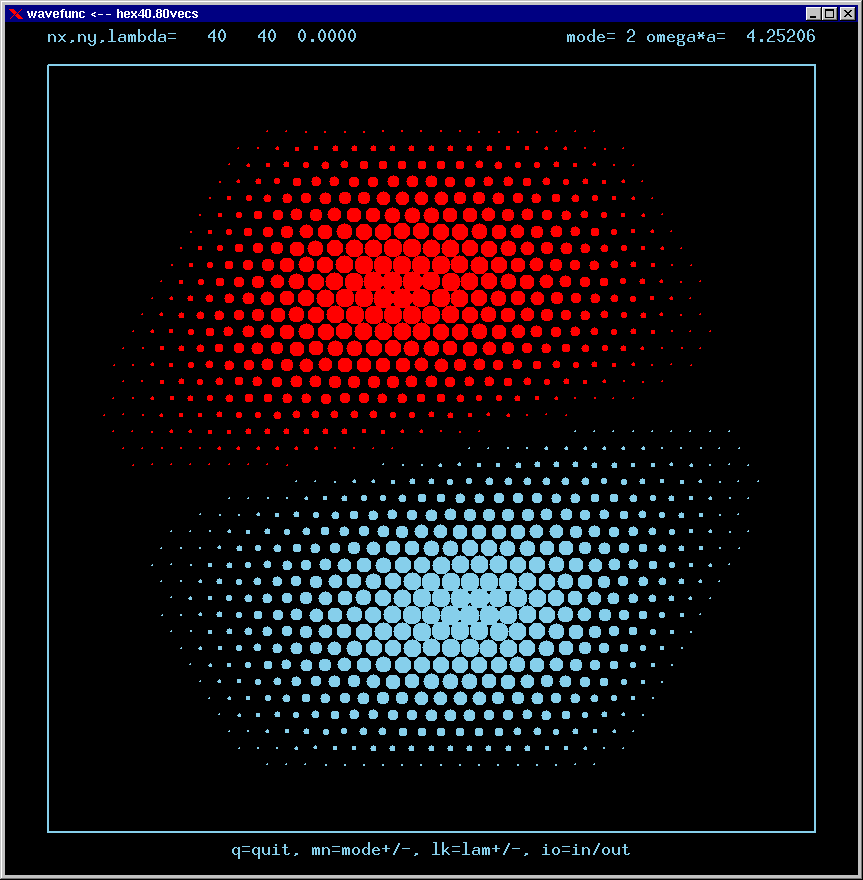
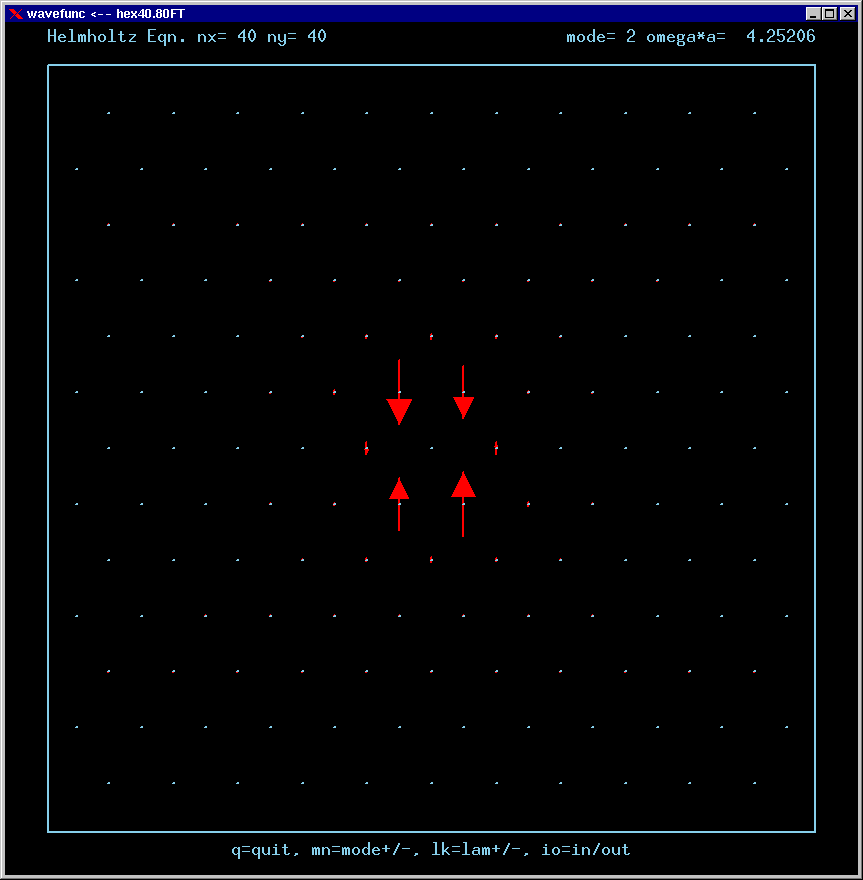
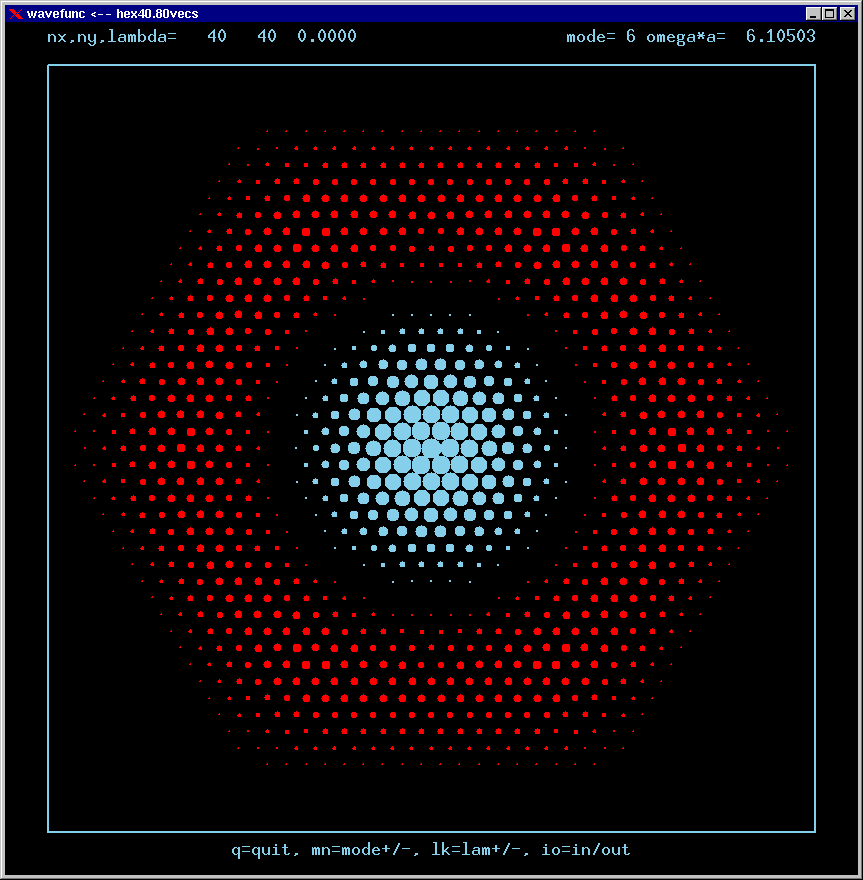
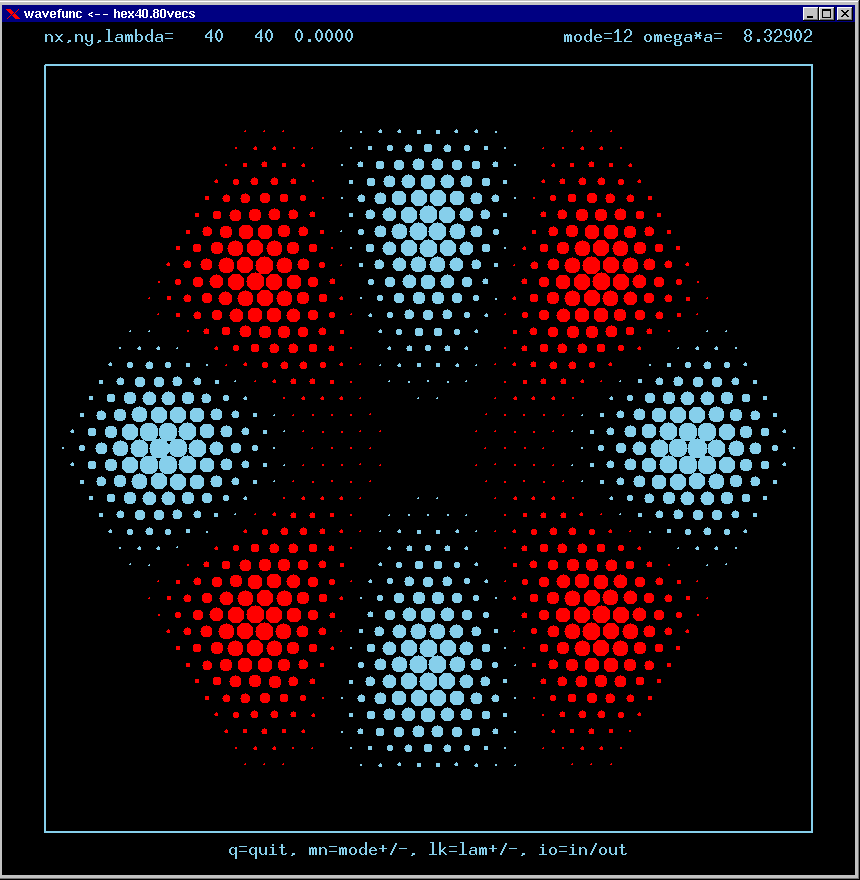
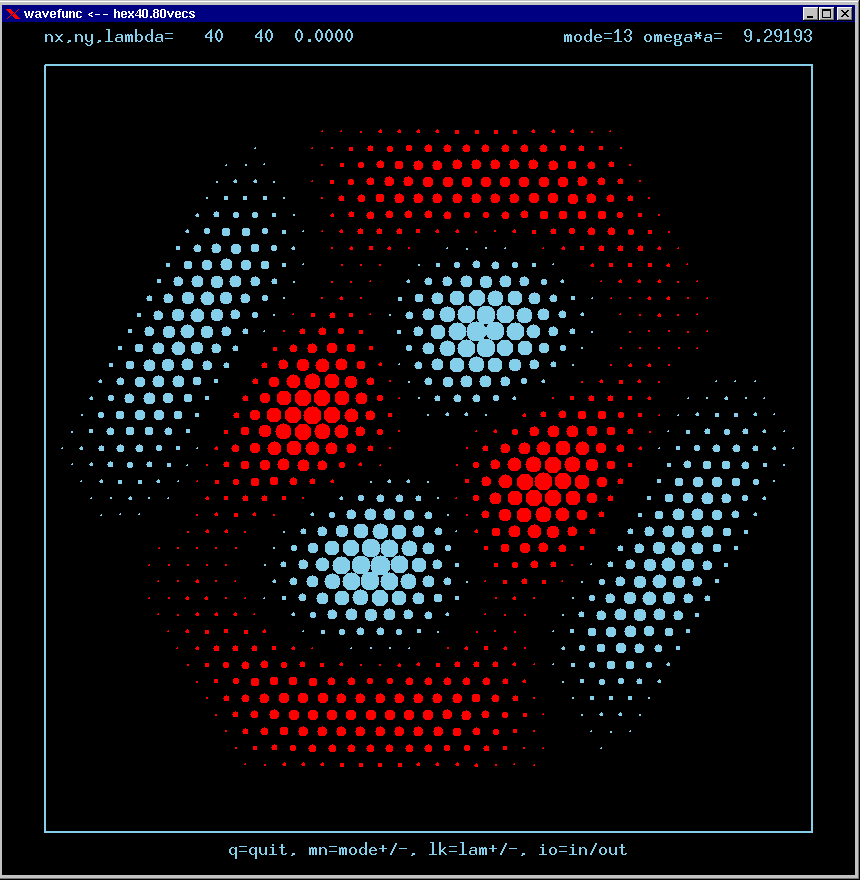
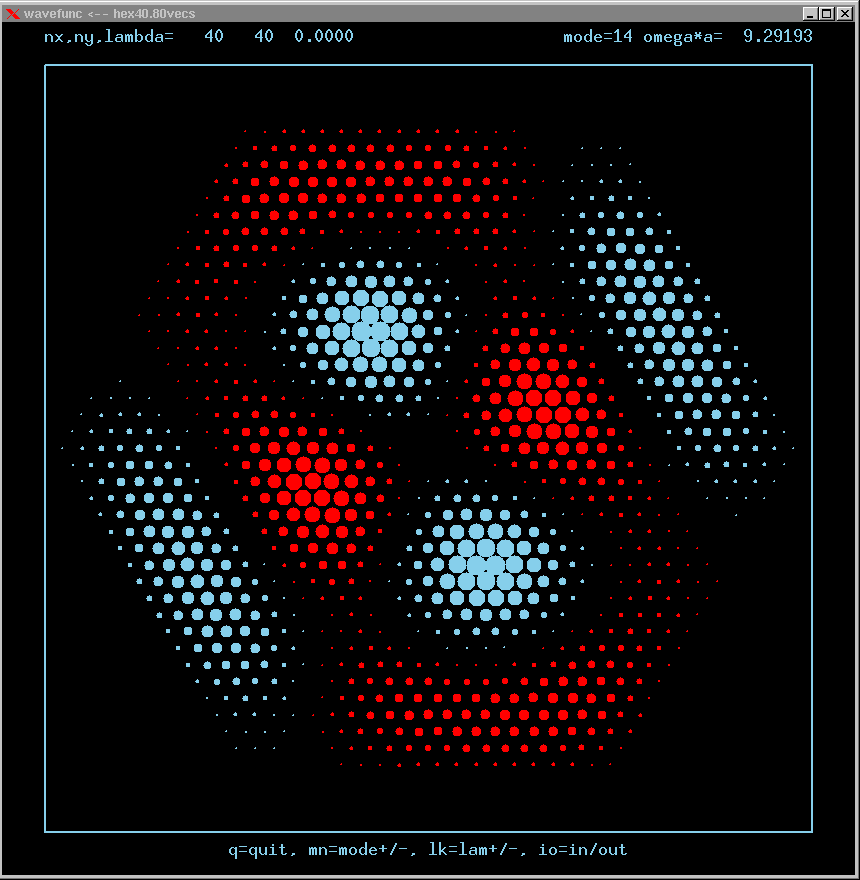
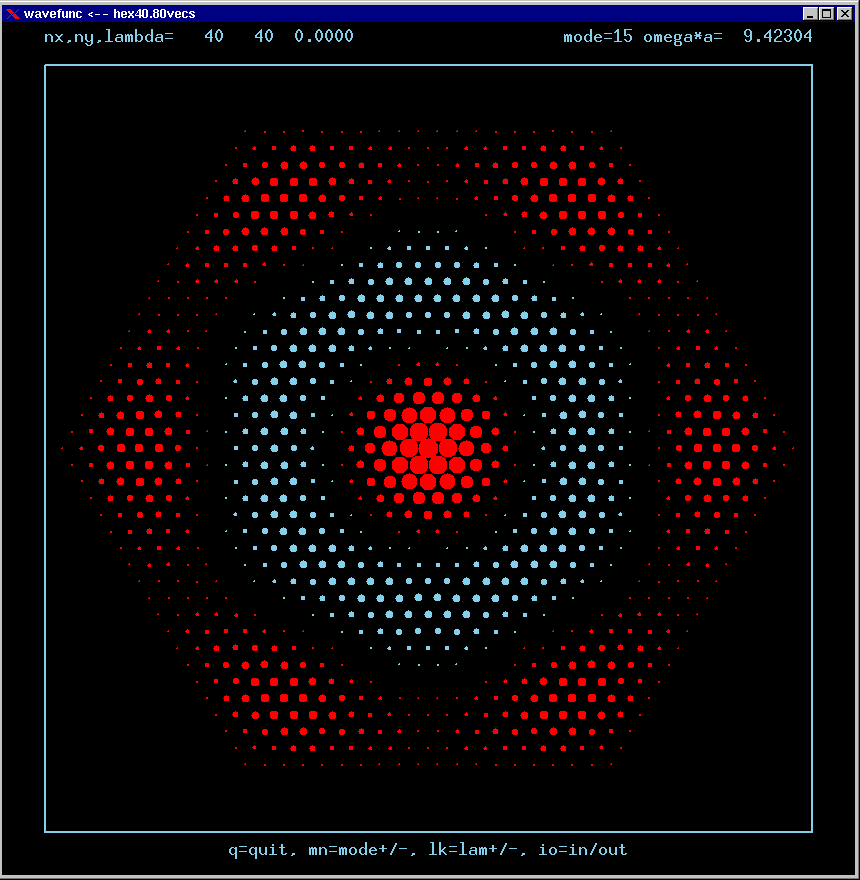
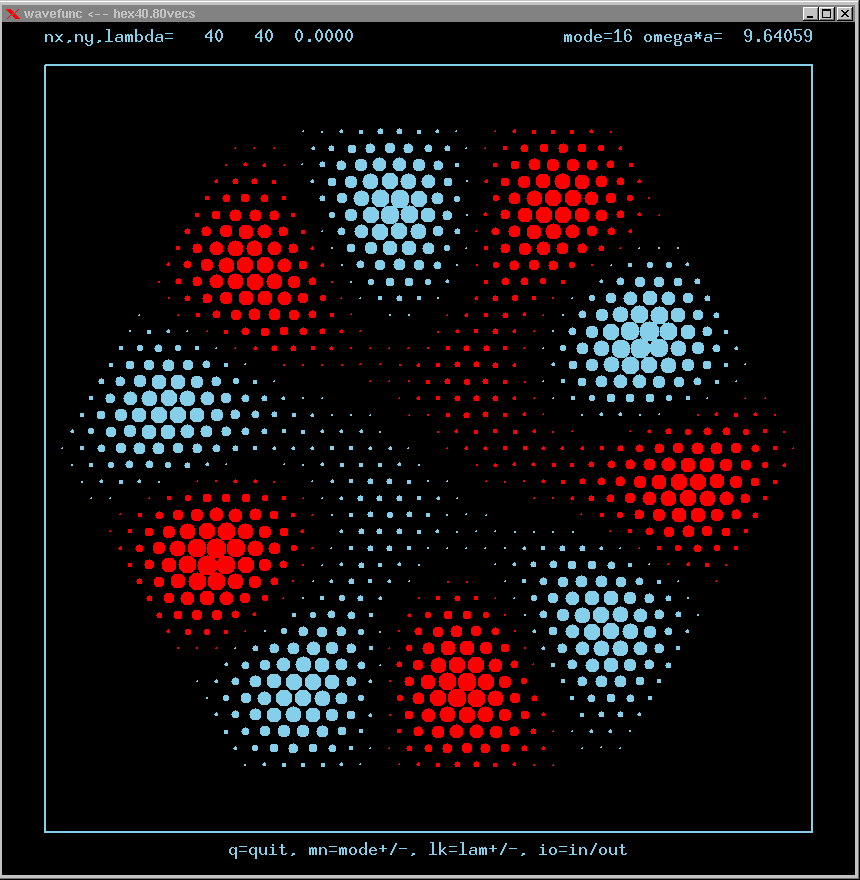
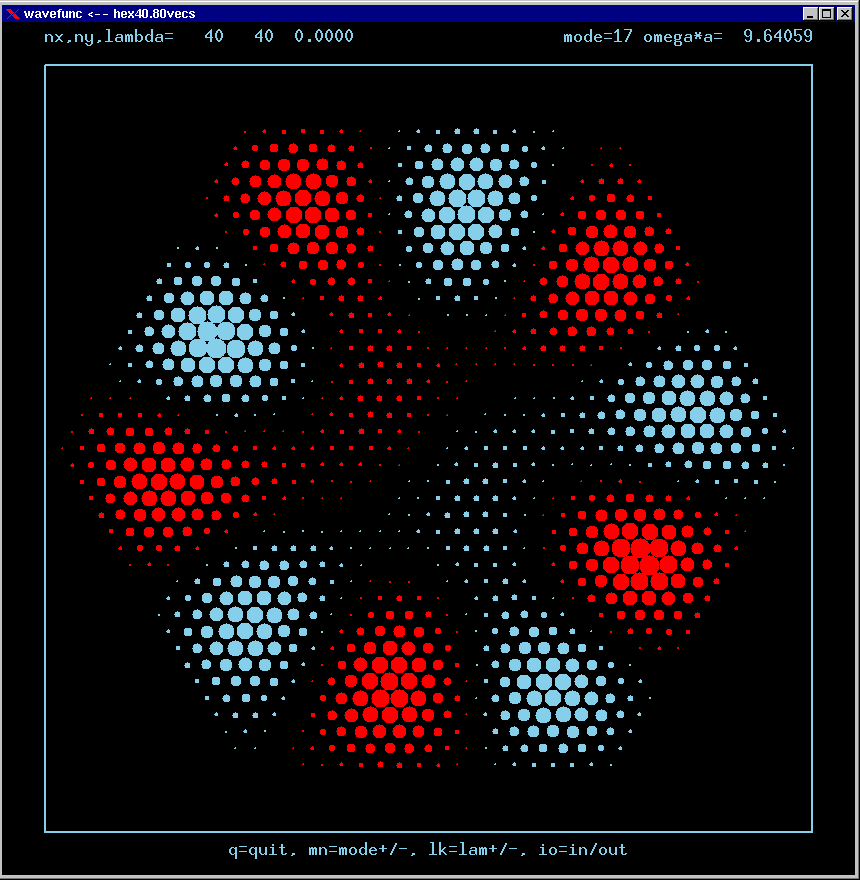
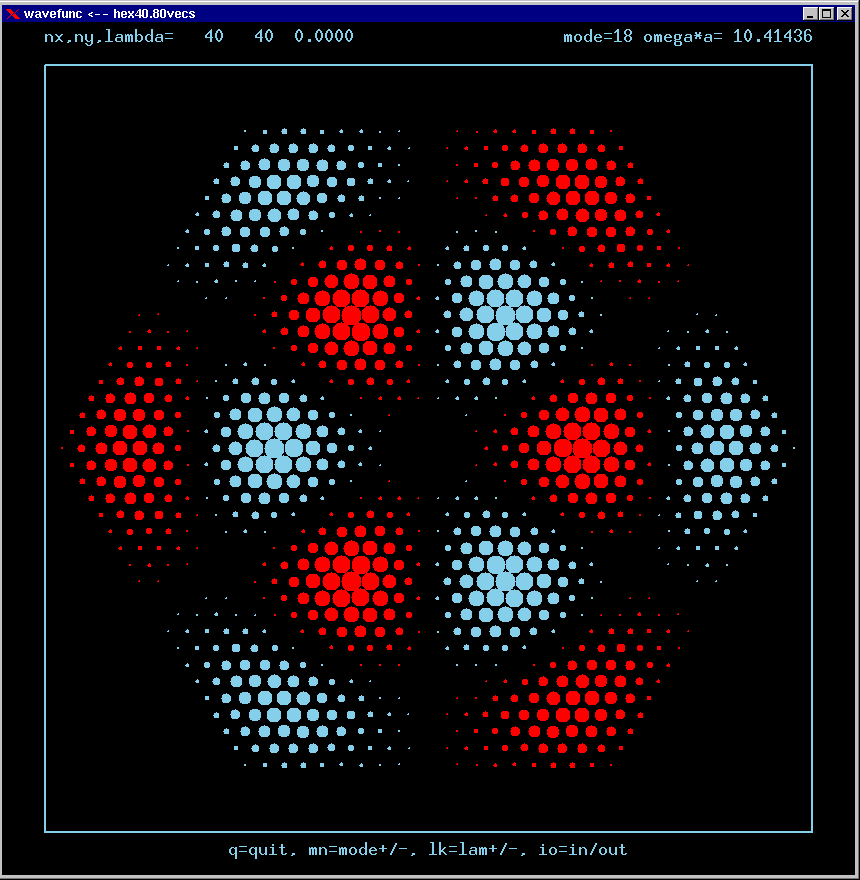
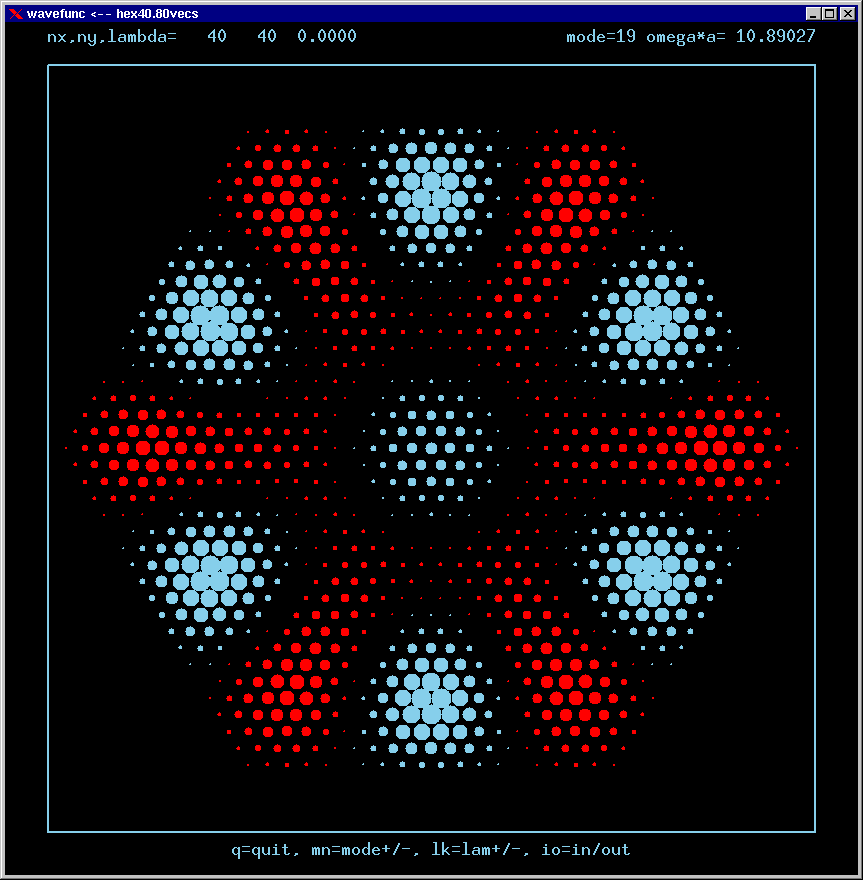
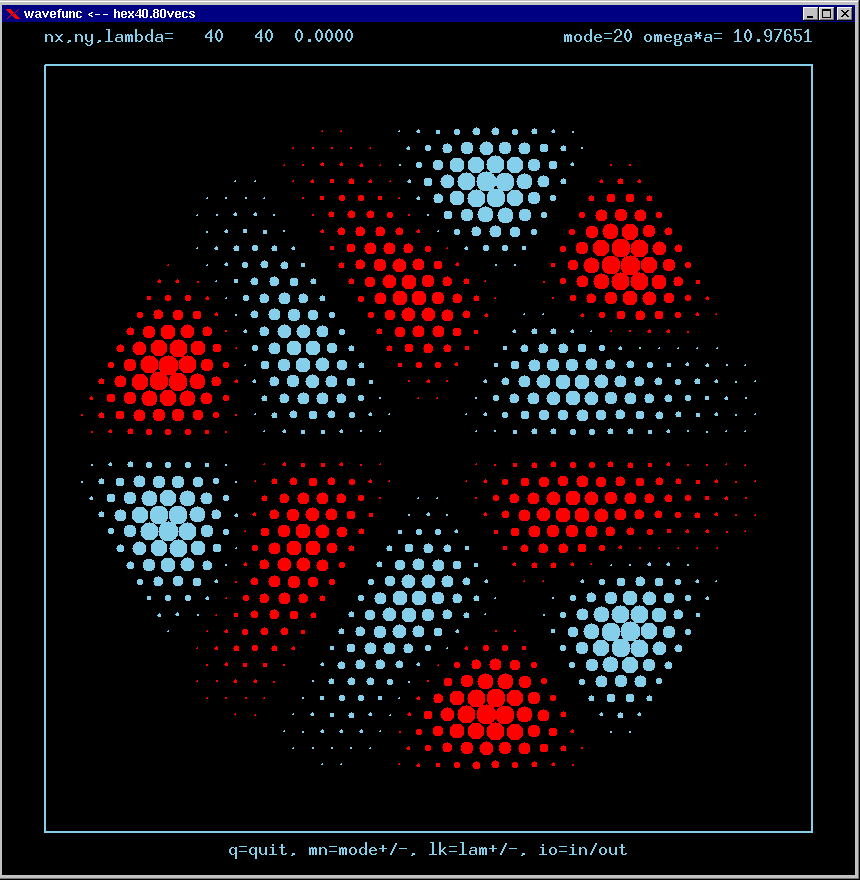
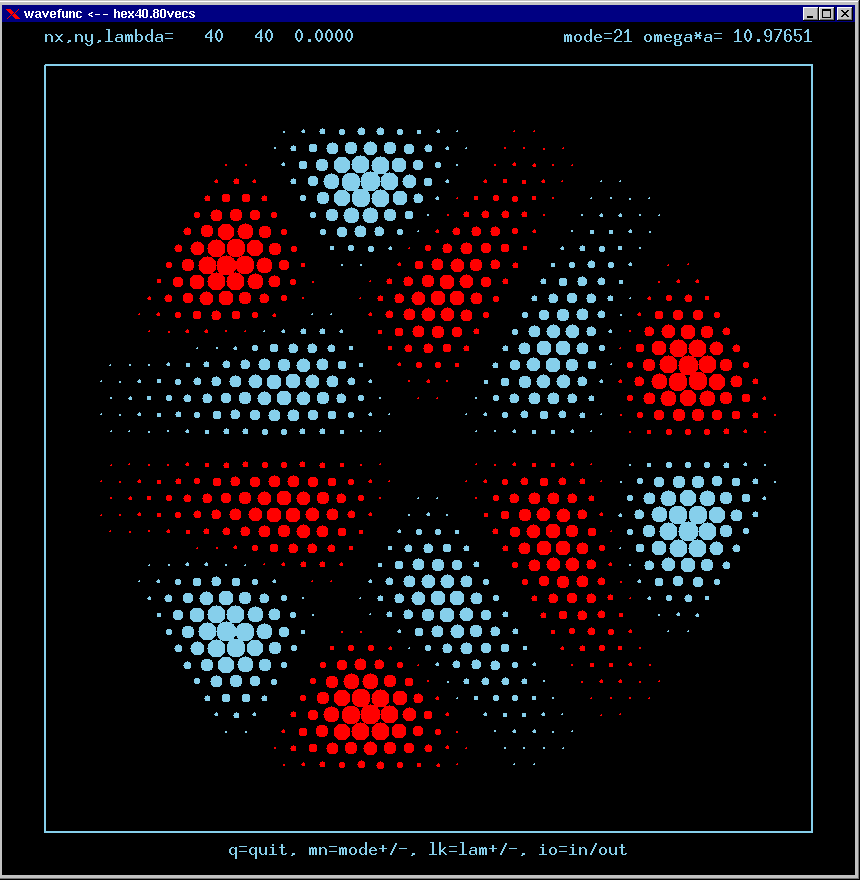
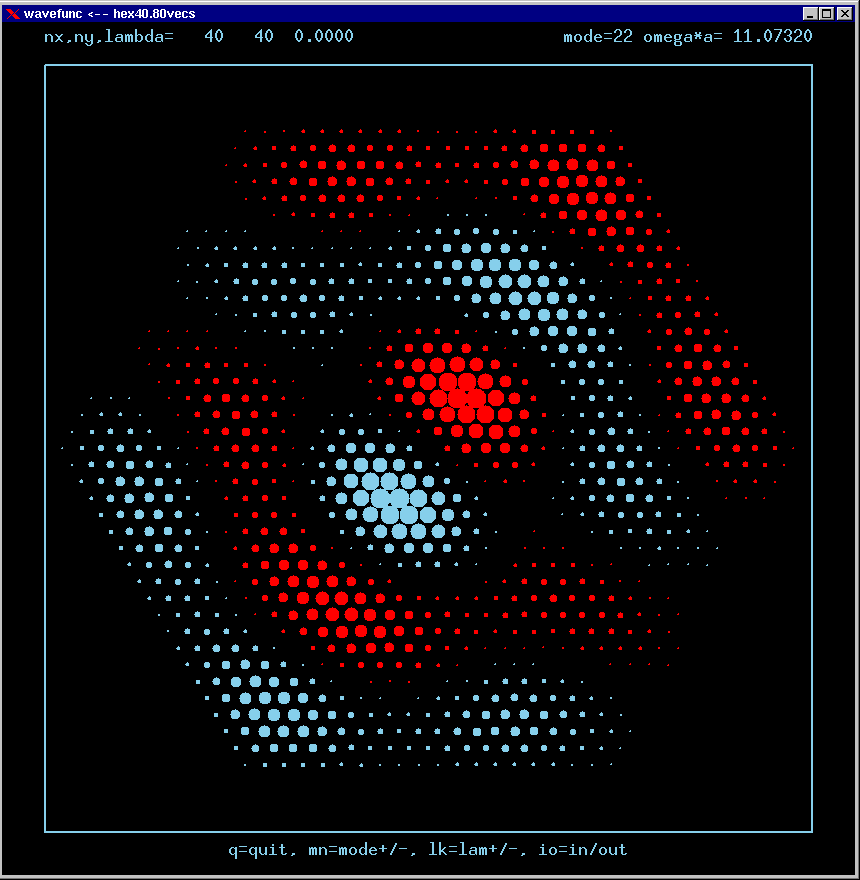
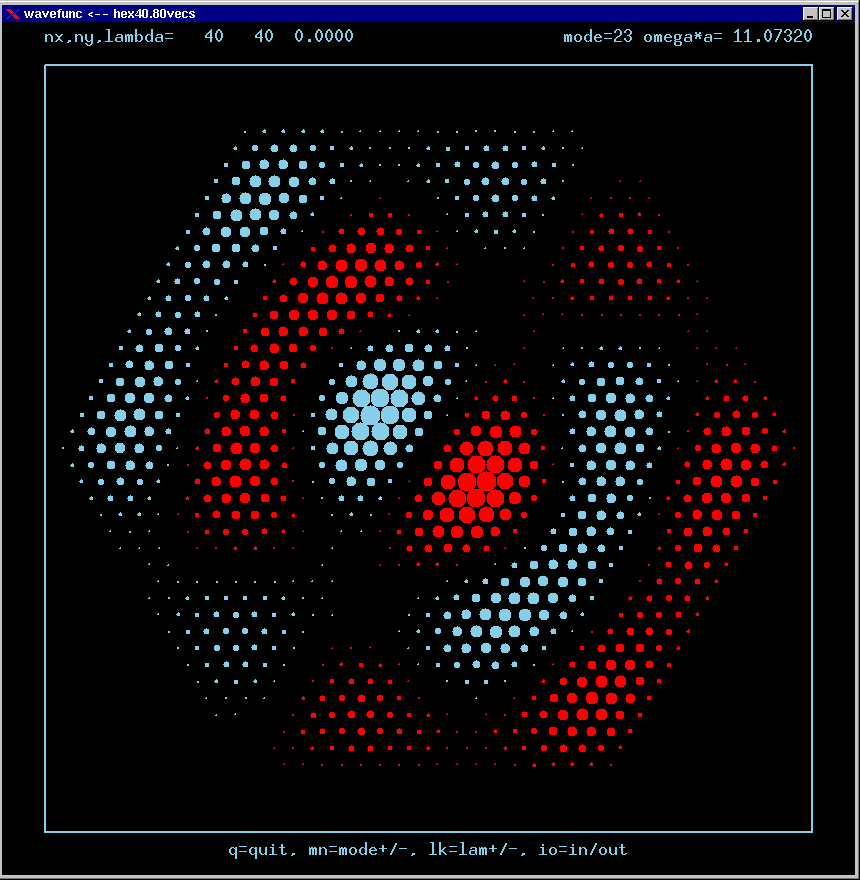
These are the real-valued wavefunctions and complex-valued Fourier Transforms of many of the lowest modes, obtained from a Gauss-Seidel numerical relaxation procedure. They are displayed on a grid for convenience; the wavefunction magnitude at each position is proportional to the symbol size there. Red is positive, blue is negative wavefunction value.
Modes are labelled only by the sequence number in which they were found, starting from the lowest frequency and working upwards in the spectrum. Some modes are single or nondegenerate. Other modes are doubly degenerate (having the same frequency as another independent mode of vibration). Certain modes are simply the analytic repetition of the modes of an equilateral triangular cavity repated over the 6 triangles making up the hexagon!
The dimensionless frequency numbers on the plots are actually omega*a*n/c, where n is the index of refraction inside the cavity, and c is the speed of light in vacuum.
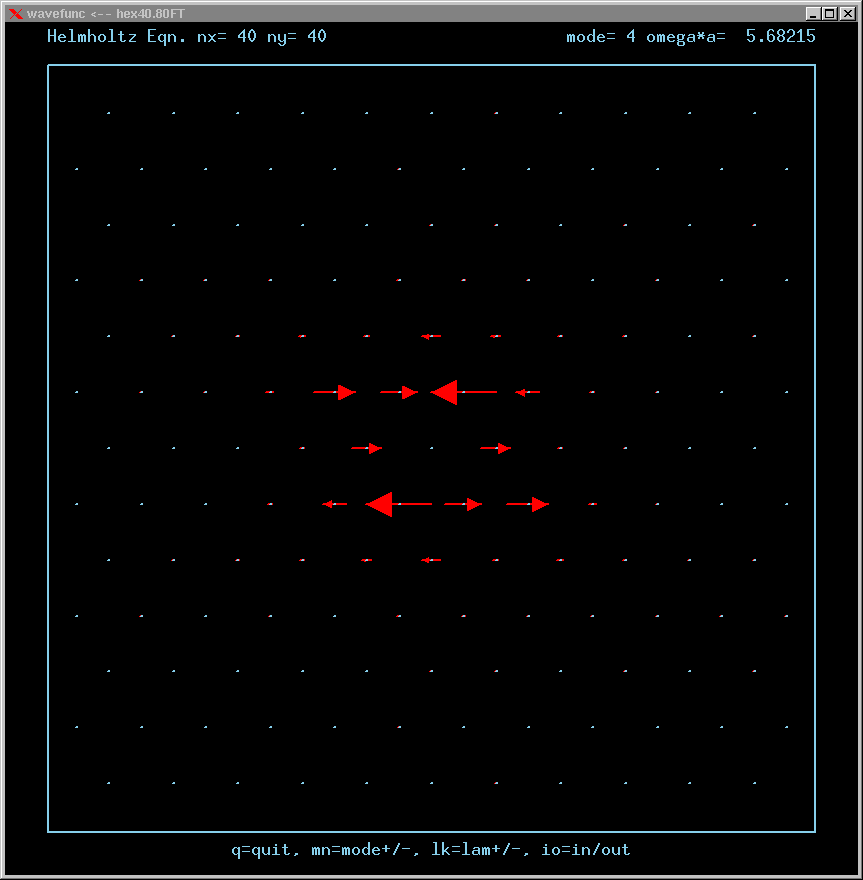
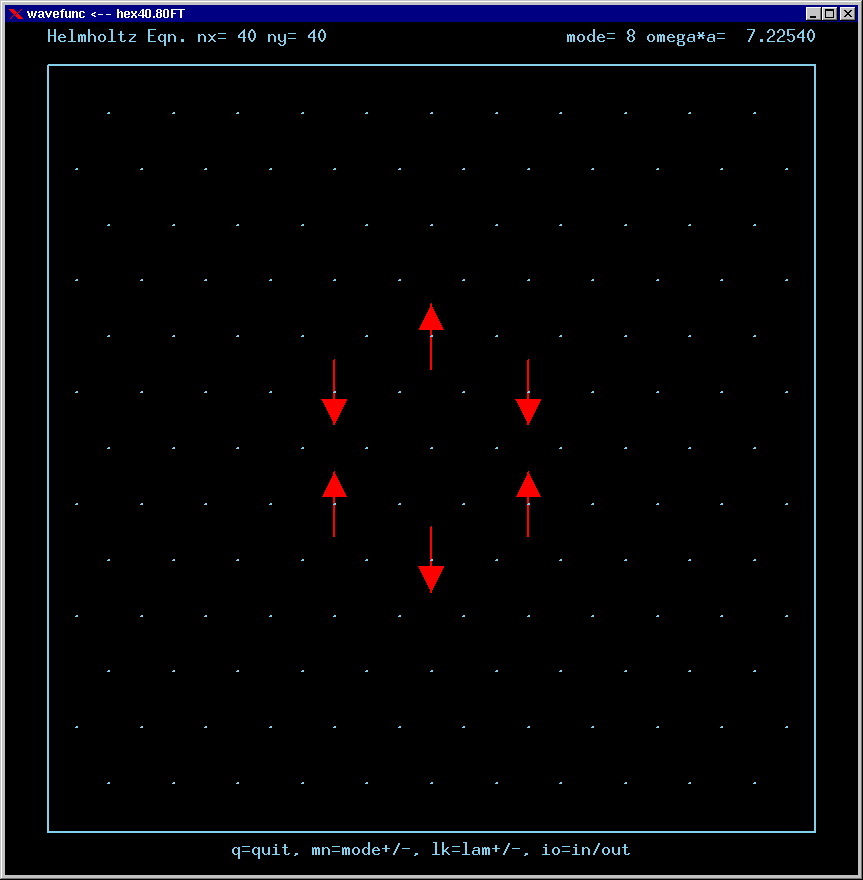
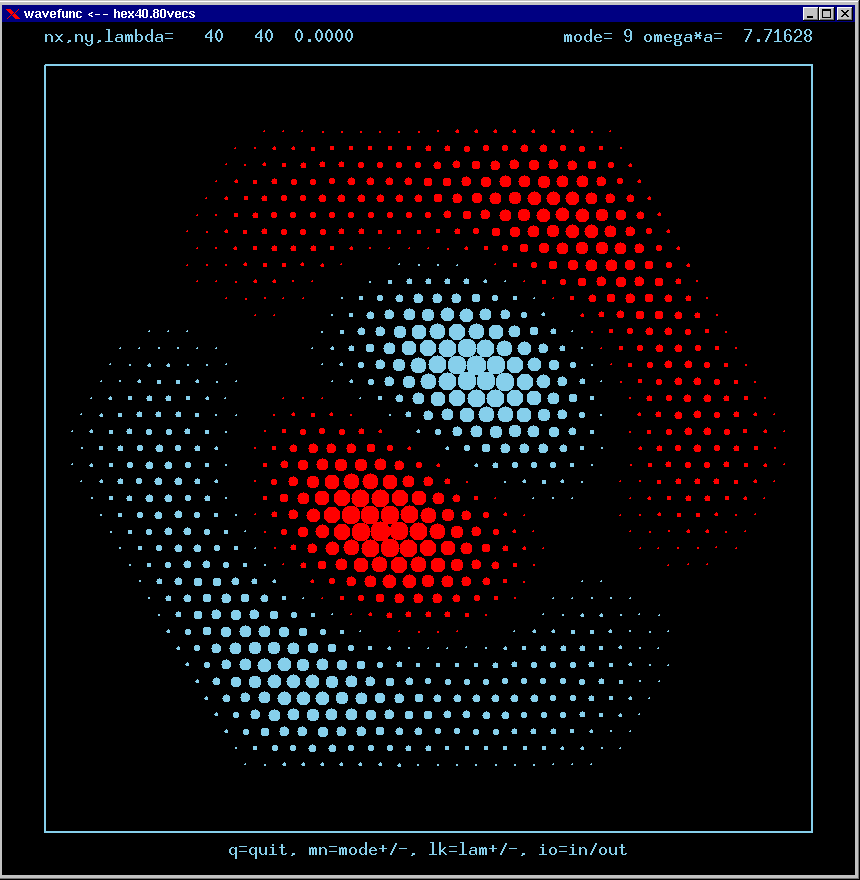
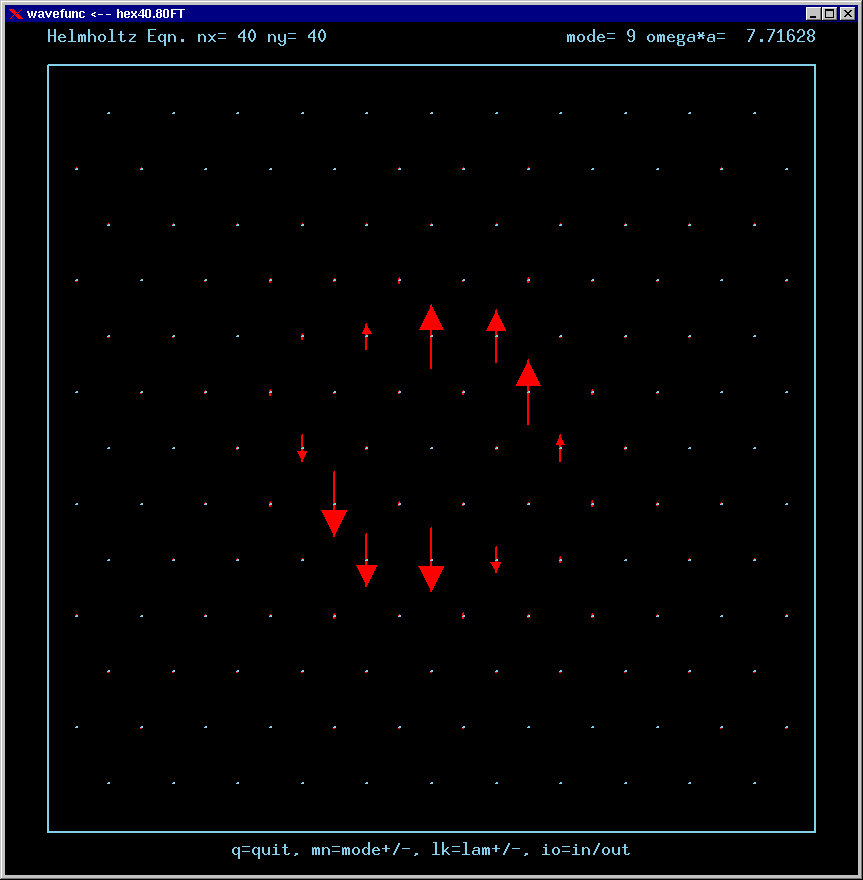
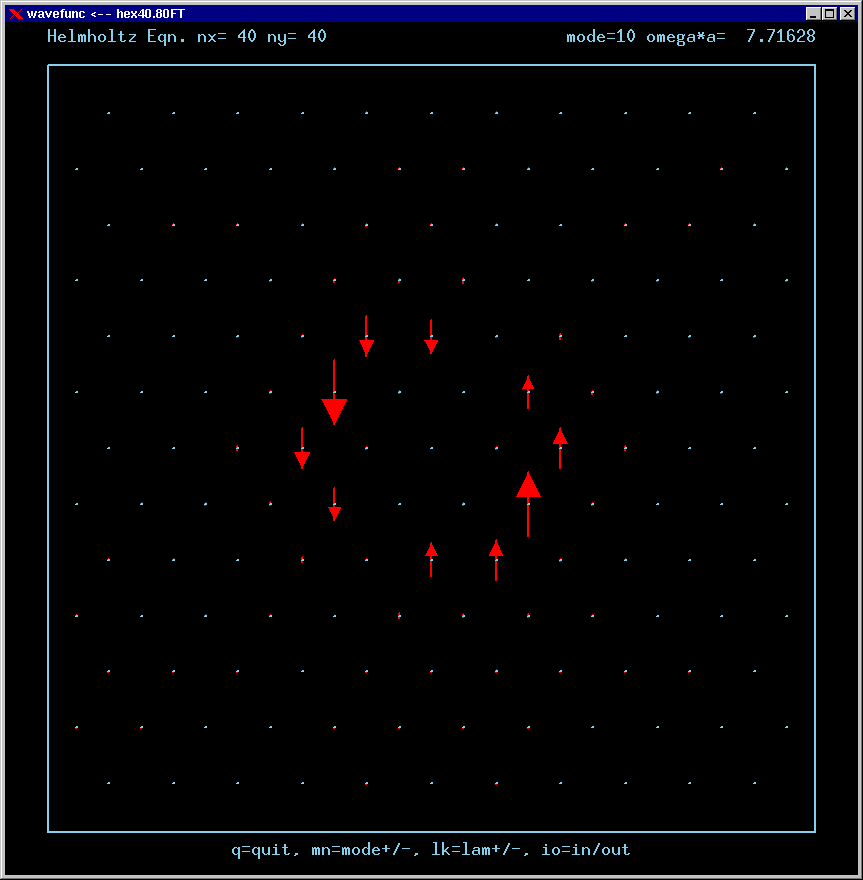
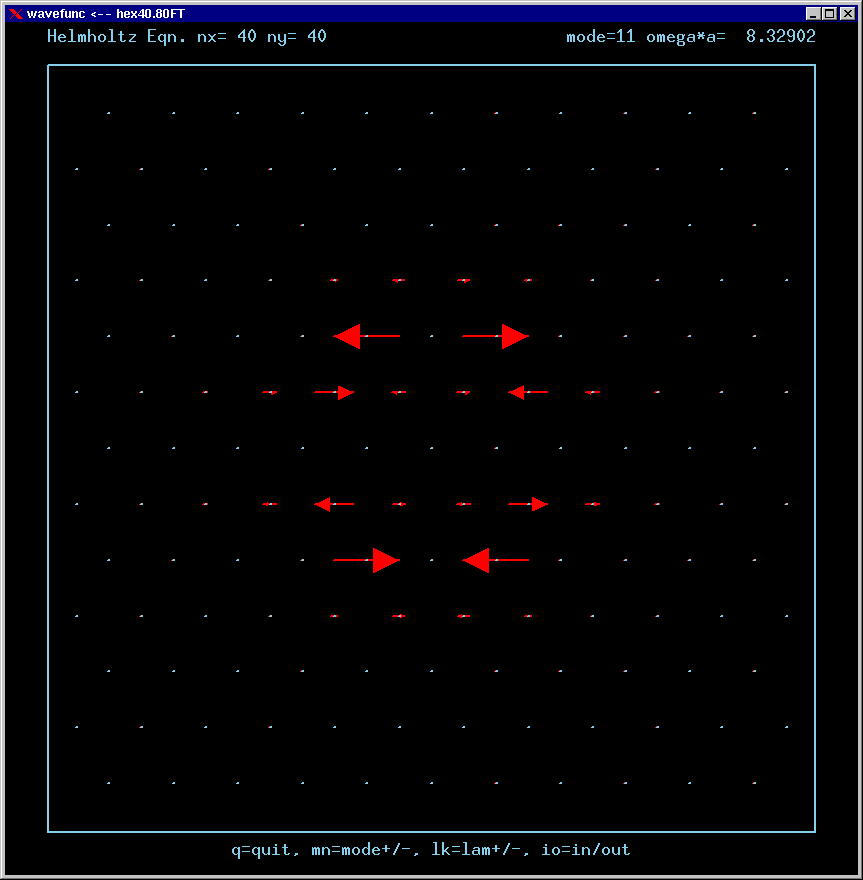
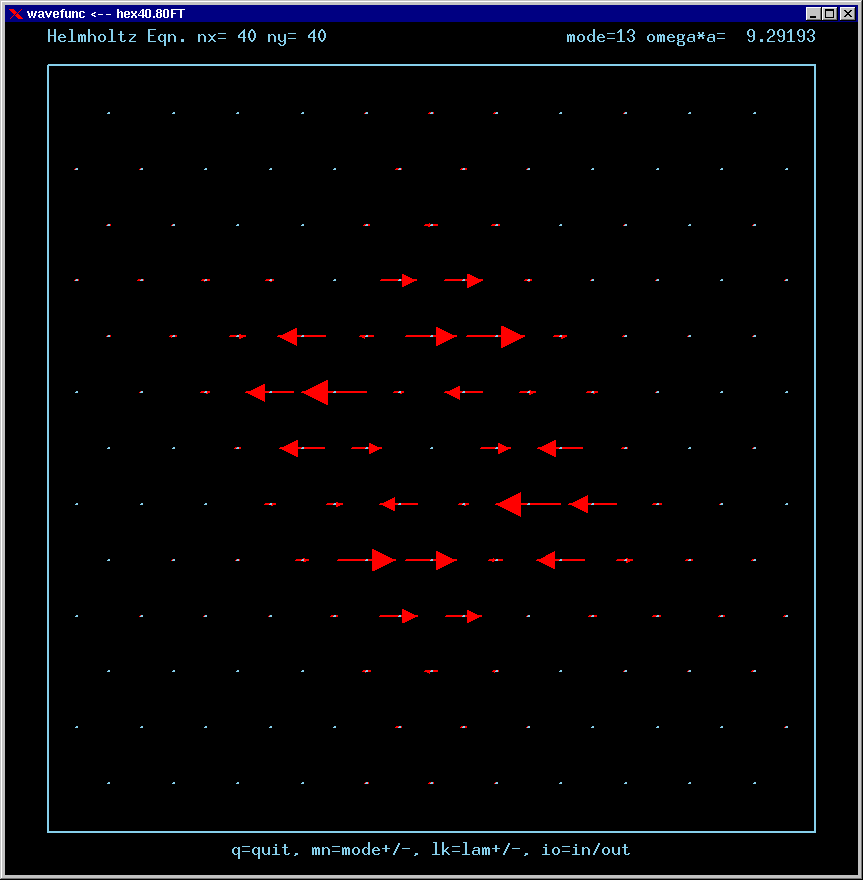
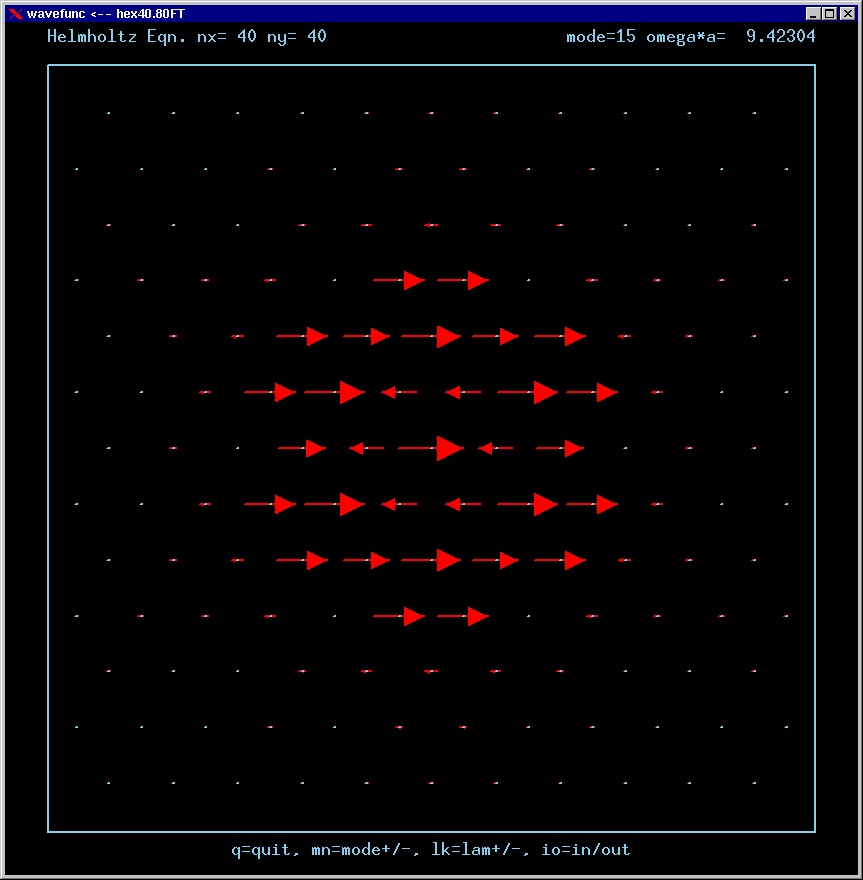
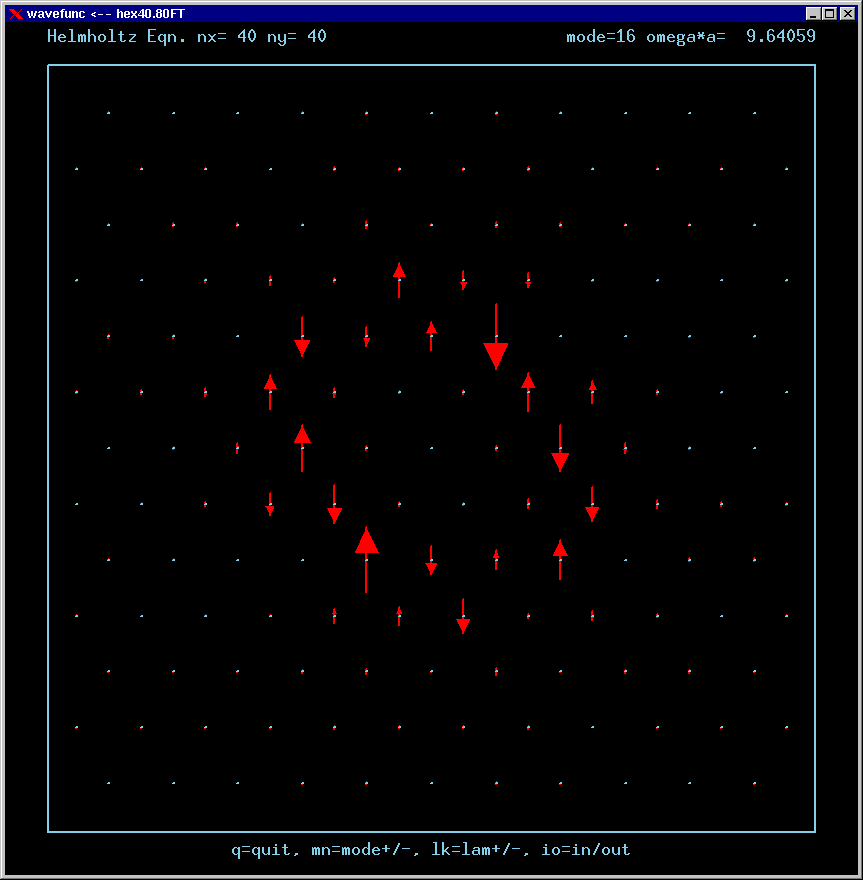
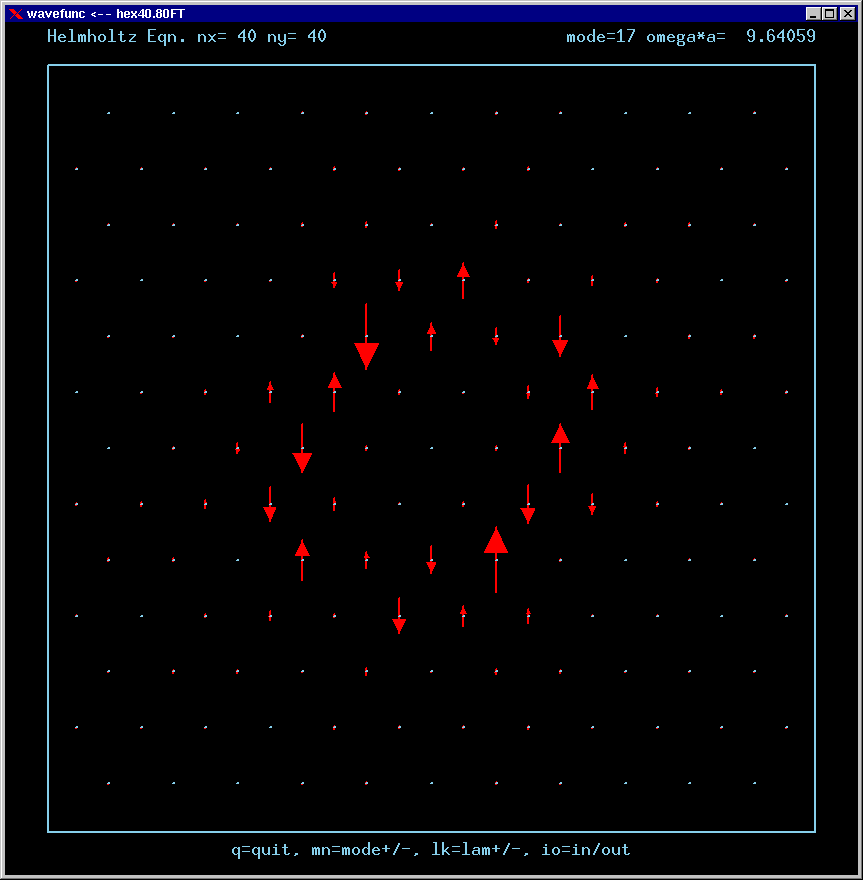
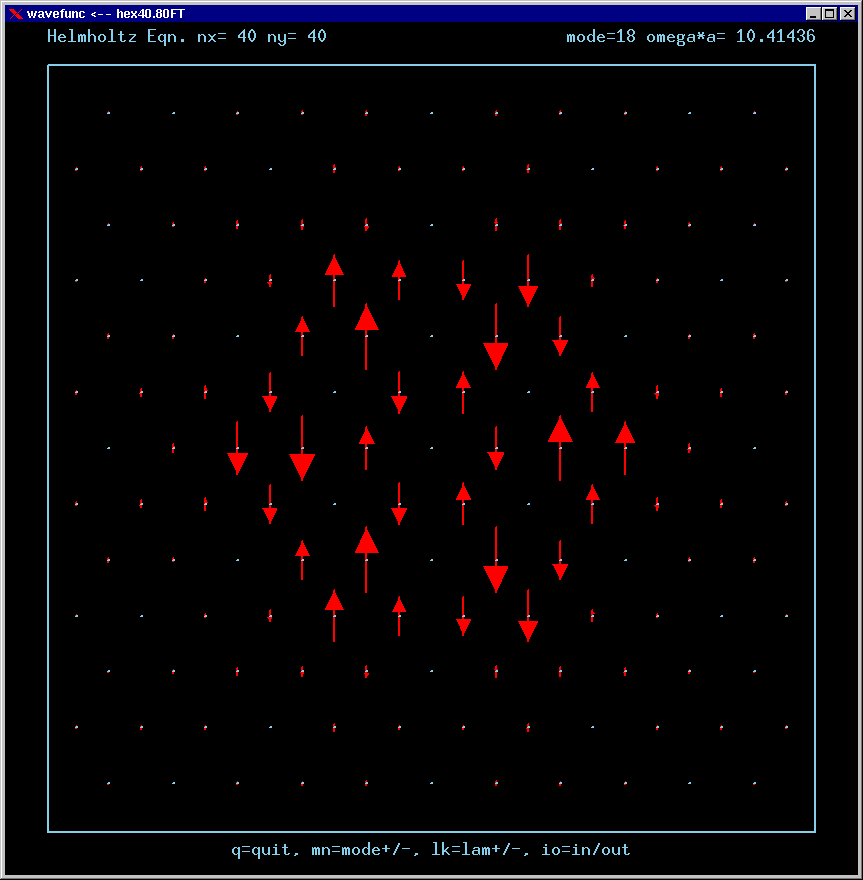
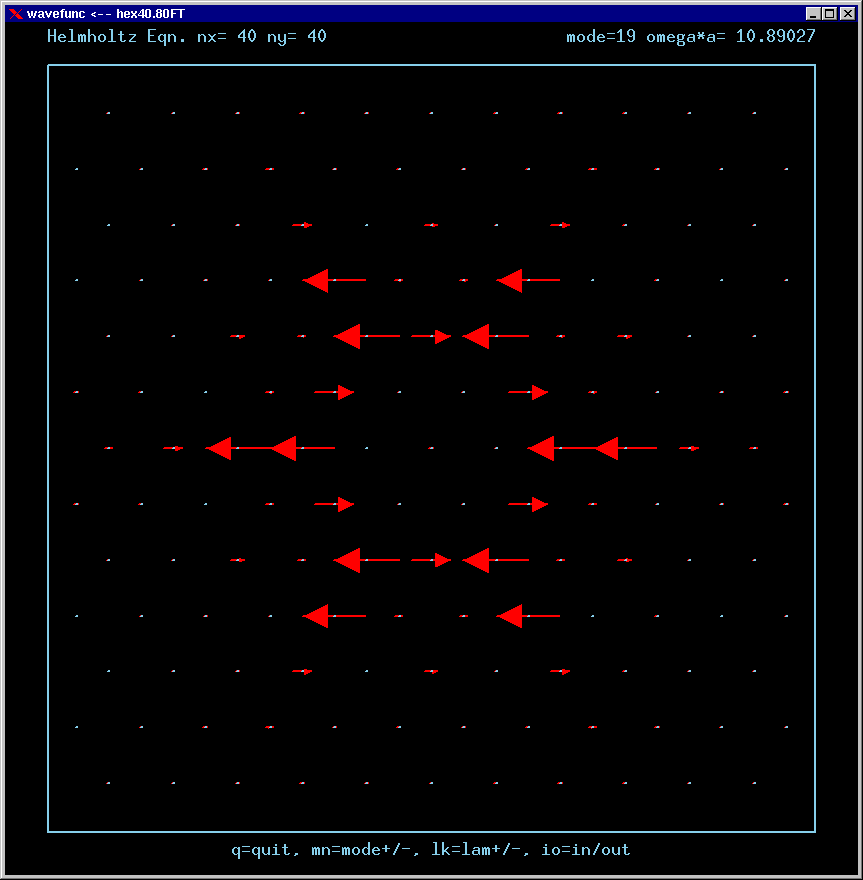
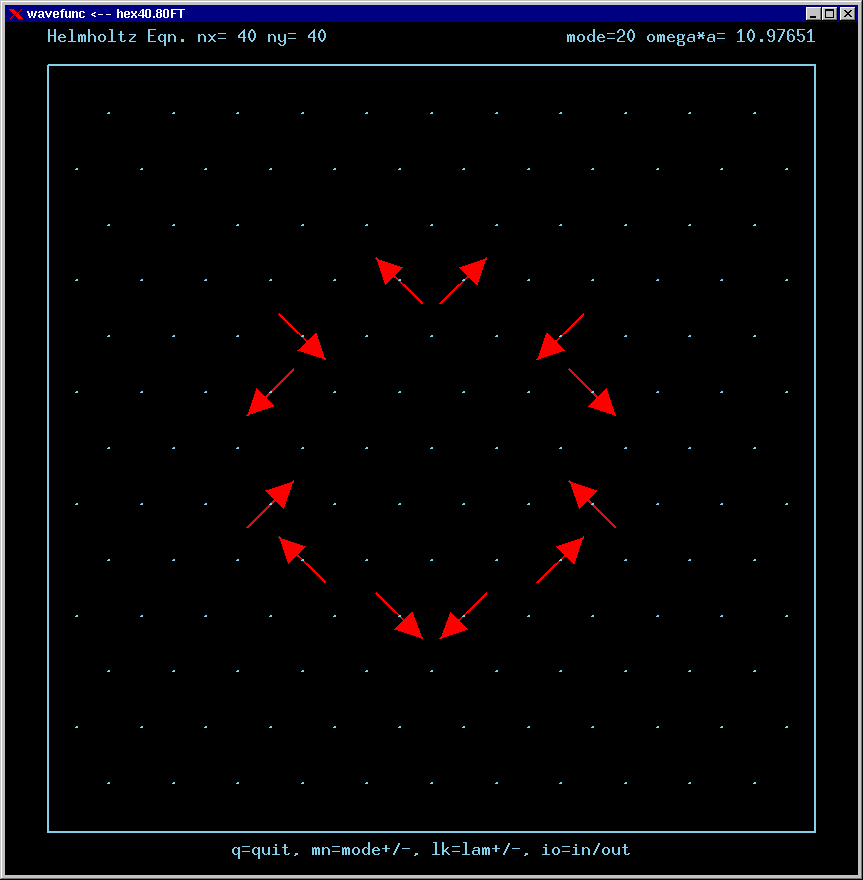
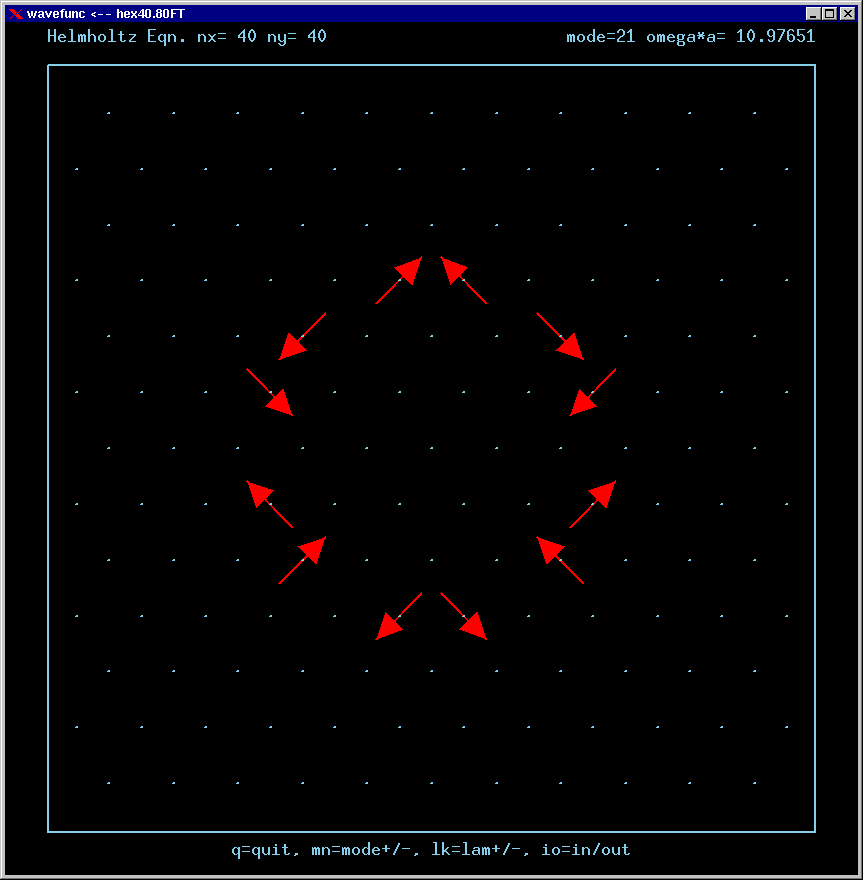
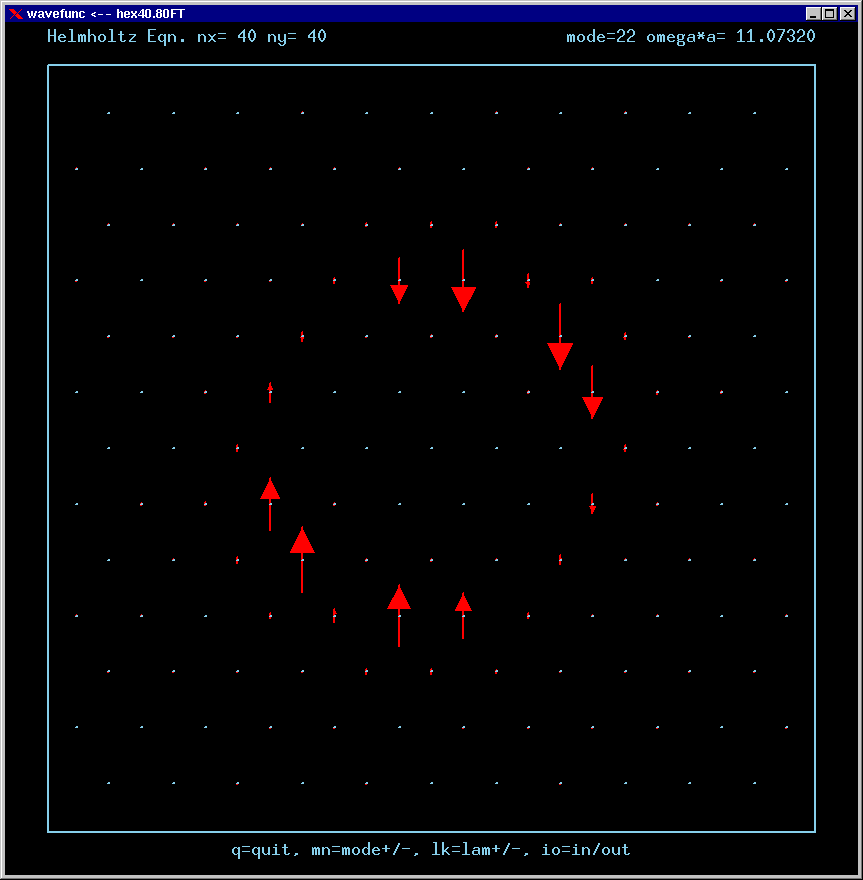
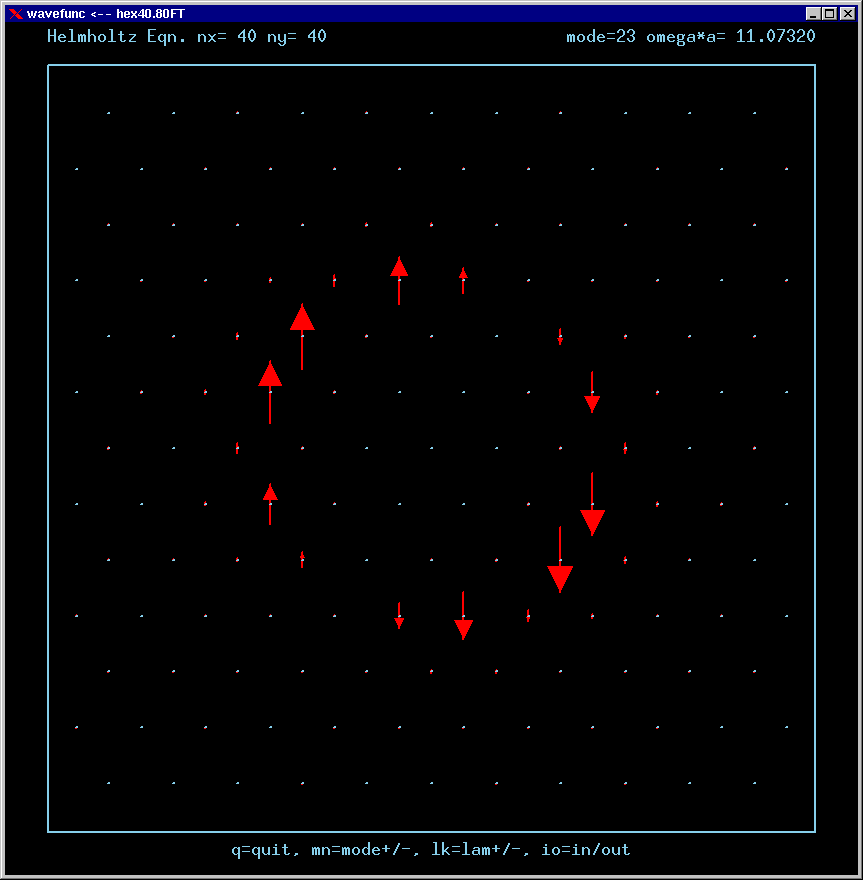
For the Fourier Transforms, the arrows represent the magnitude and phase of the Fourier amplitude at a chosen wavevector. The spacing of the grid points in Fourier space is 4*pi/3a. The entire Brillouin zone is hexagonally shaped. The wavevector (kx,ky)=(0,0) is the point at the center of the diagram. Only the important inner part of the BZ is shown in most of these figures.
A mode can be confined by total internal reflection as long as there is no FT amplitude propagating normal to any boundary. For example, applying this reasoning to the upper horizontal boundary, a mode cannot be confined if there is FT amplitude along the positive vertical axis of the FT diagram.
If a mode has no FT amplitudes anywhere near normal incidence to any boundary, it is called a strong TIR state .
If a mode has no FT amplitudes at normal incidence to the boundaries, but does have amplitudes at arbitrarily small incident angles, then it is called a weak TIR state.
Click image for a postscript file of that figure.
Mode 1 (ground state, nondegenerate) wavefunction and zoomed Fourier transform:


Mode 2 (degenerate with 3) and its FT:
Mode 3 (degenerate with 2) and its FT:
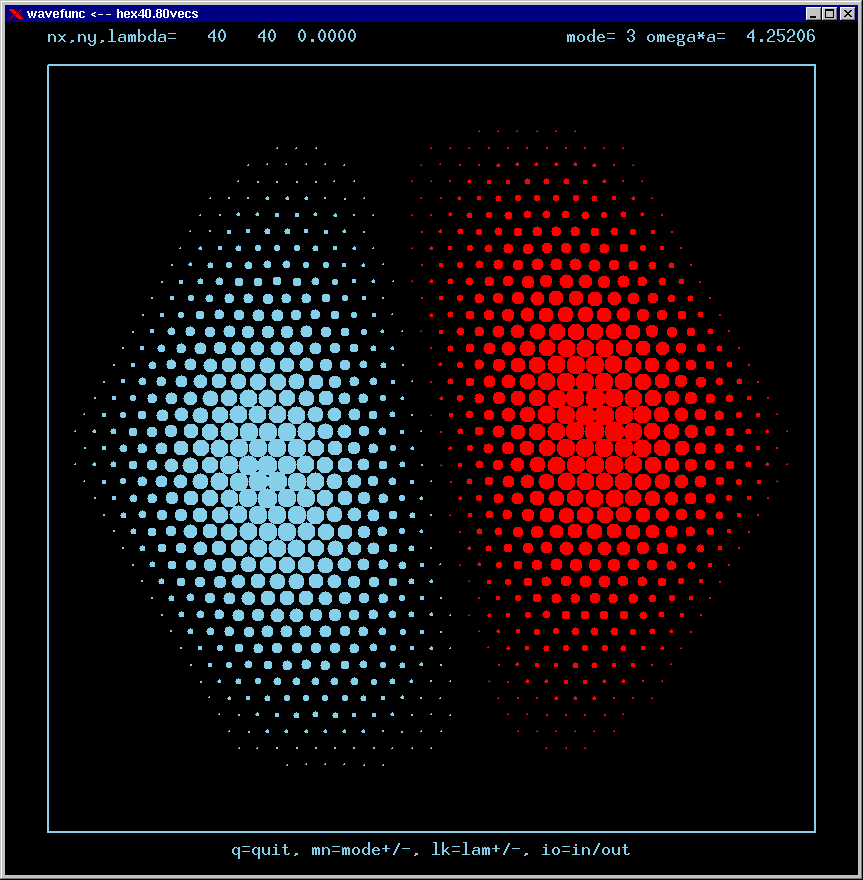

Mode 4 (degenerate with 5) and its FT:
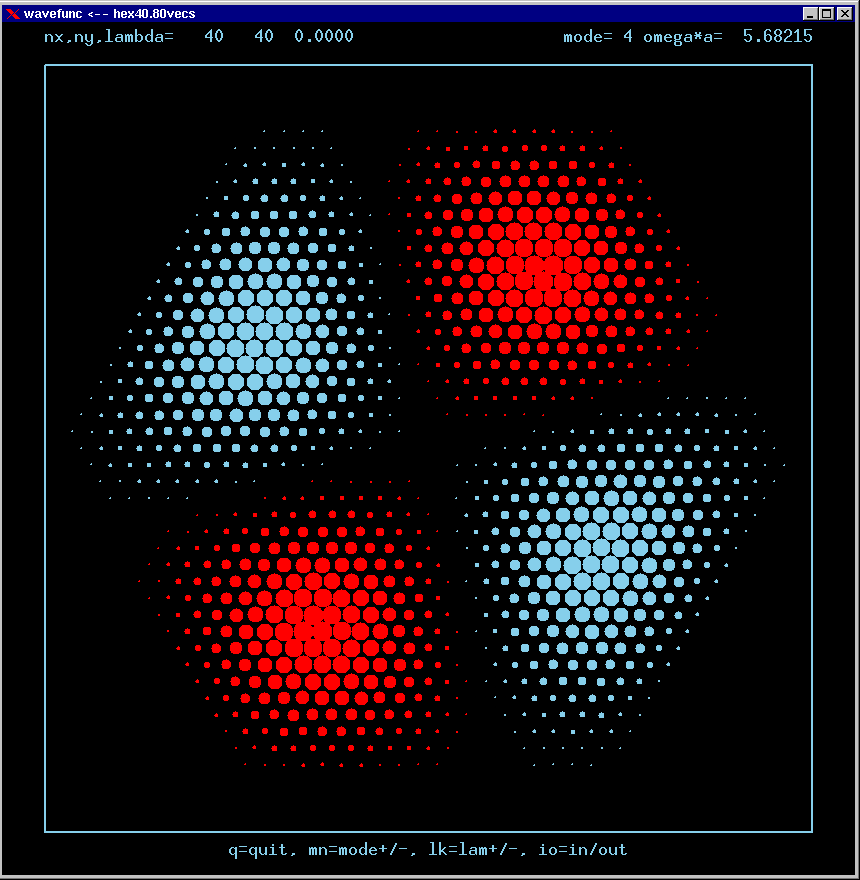
Mode 5 (degenerate with 4) and its FT:


Mode 6 (nondegenerate) and its FT:
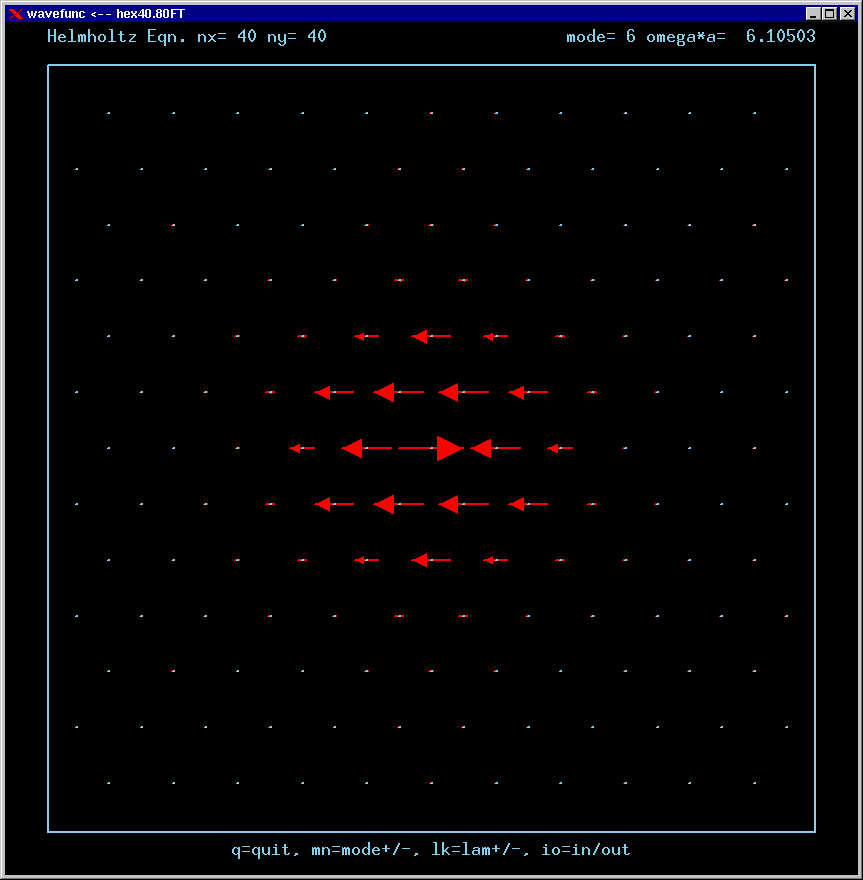
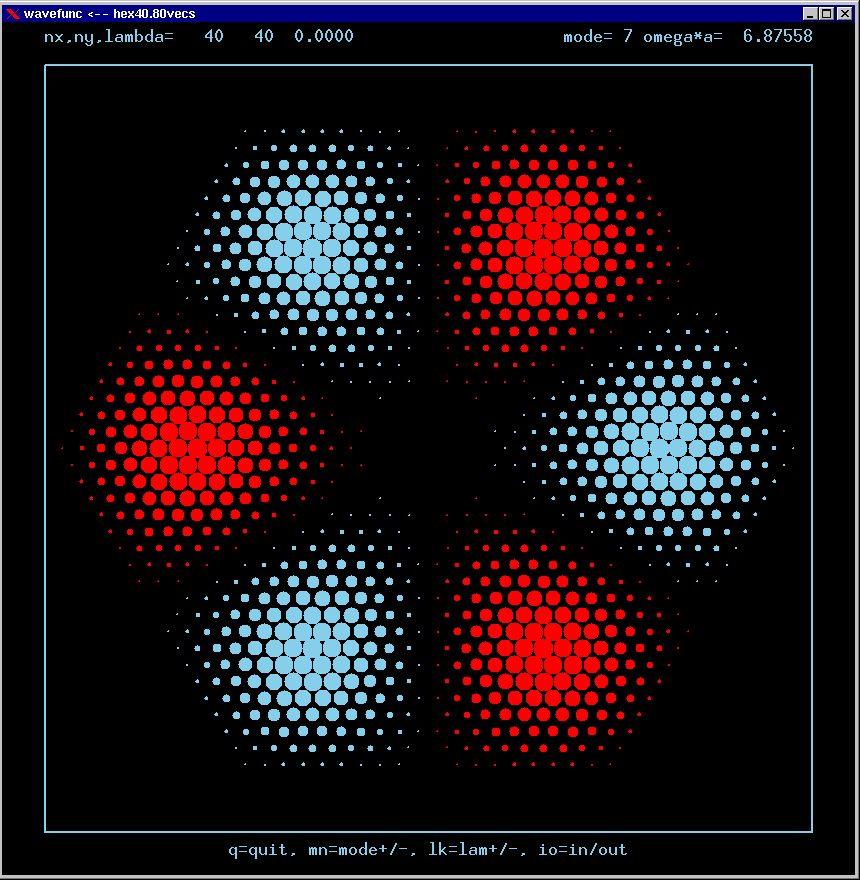
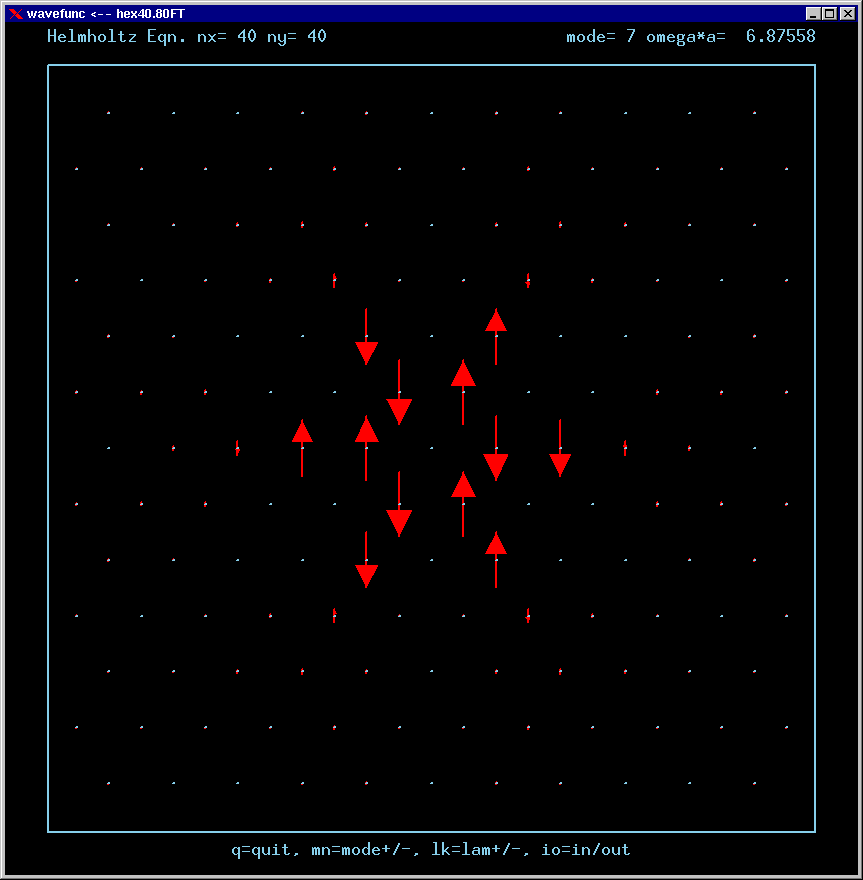
Mode 7 (nondegenerate, weak TIR) and its FT:
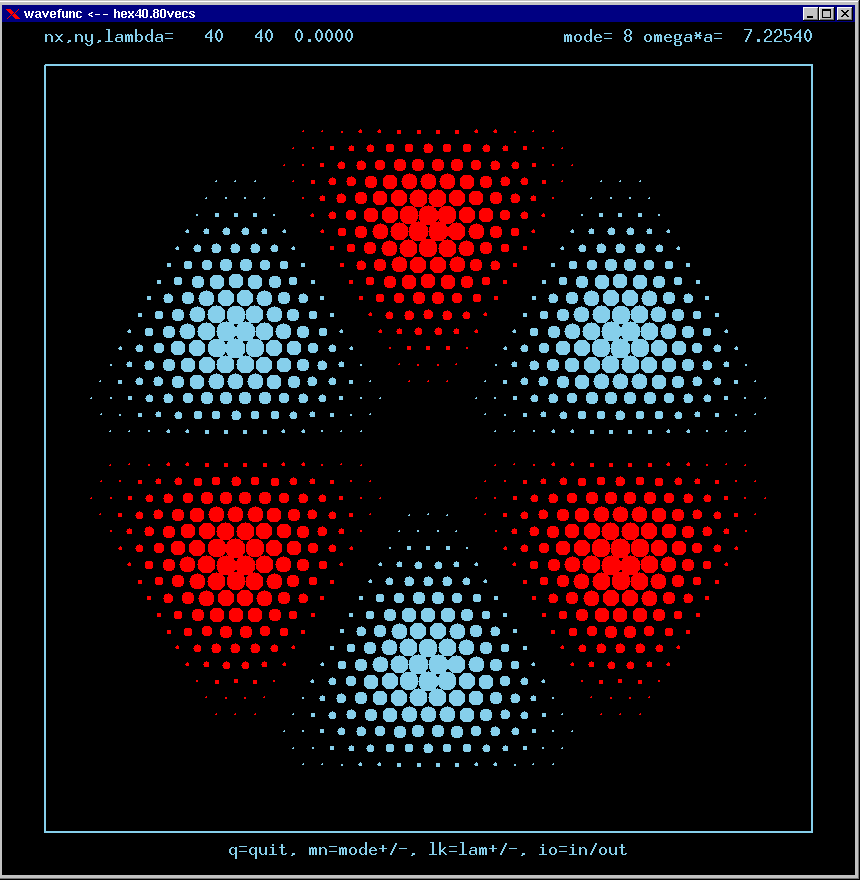
Mode 8 (nondegenerate, m=0, n=2 triangle state, NO TIR) and its FT:
Mode 9 (degenerate with 10) and its FT:
Mode 10 (degenerate with 9) and its FT:

Mode 11 (degenerate with 12) and its FT:

Mode 12 (degenerate with 11) and its FT:
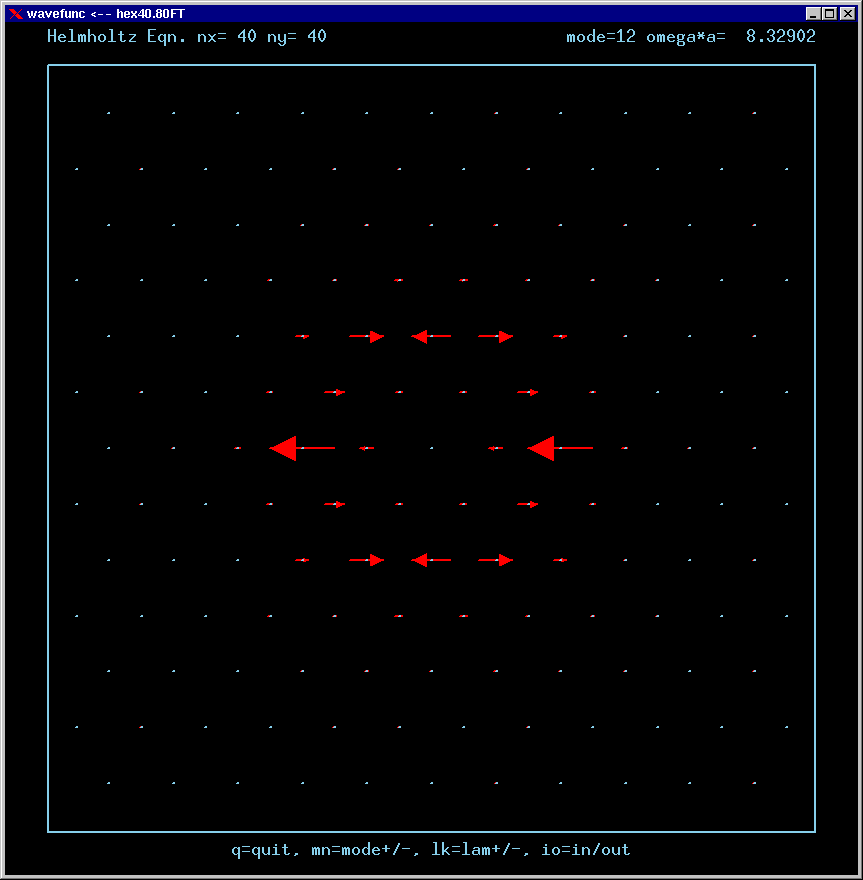
Mode 13 (degenerate with 14) and its FT:
Mode 14 (degenerate with 13) and its FT:

Mode 15 (nondegenerate) and its FT:
Mode 16 (degenerate with 17) and its FT:
Mode 17 (degenerate with 16) and its FT:
Mode 18 (nondegenerate, weak TIR) and its FT:
Mode 19 (nondegenerate) and its FT:
Mode 20 (degenerate with 21, m=1, n=3 triangle state, strong TIR) and its FT:
Mode 21 (degenerate with 20, m=1, n=3 triangle state, strong TIR) and its FT:
Mode 22 (degenerate with 23) and its FT:
Mode 23 (degenerate with 22) and its FT:
Mode 24 (degenerate with 25) and its FT:
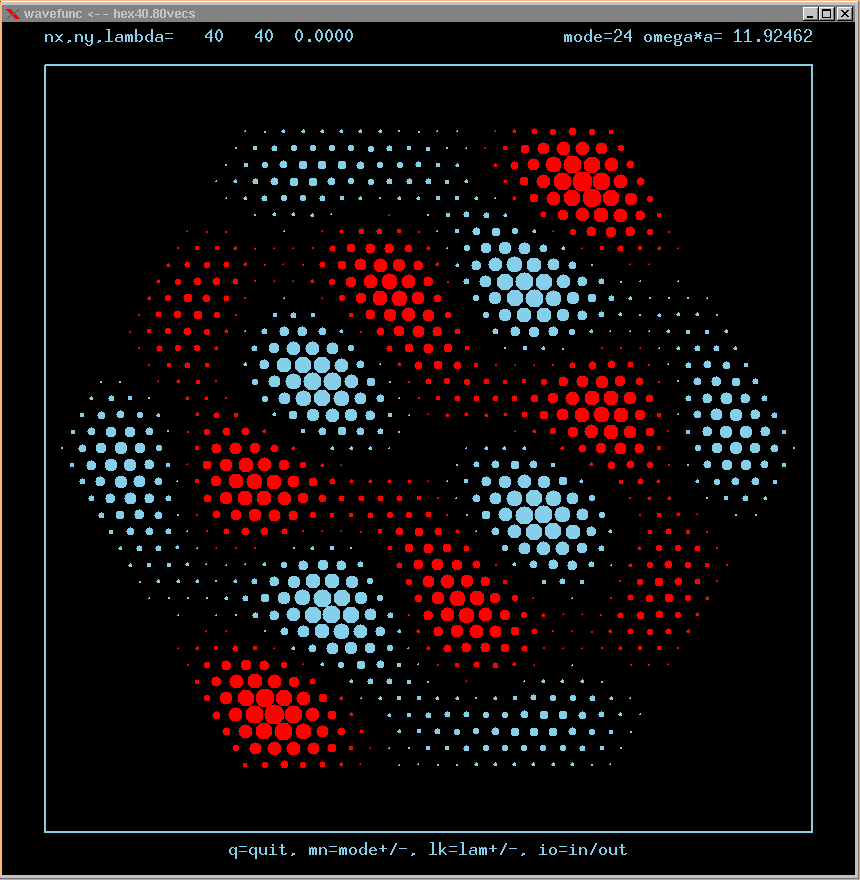
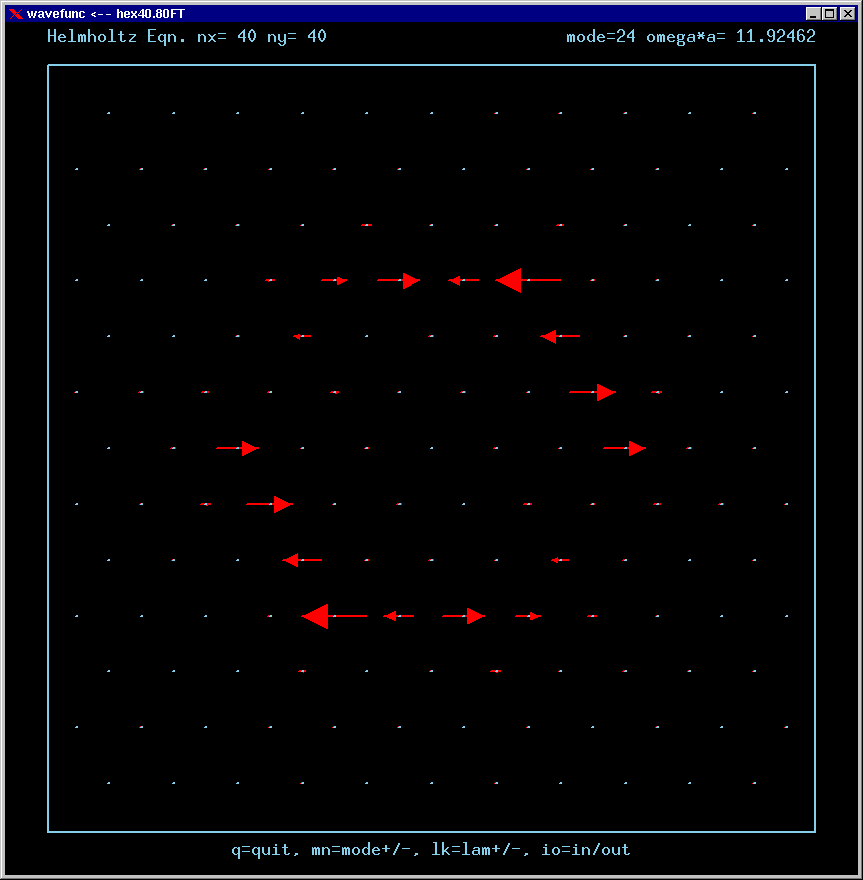
Mode 25 (degenerate with 24) and its FT: