Title

        Kurtis D. Borne

Supervisors:	Artem Rudenko: Assistant Professor of Physics
	Daniel Rolles:     Assistant Professor of Physics

Kansas State University Physics Department REU Program

 This program is funded by the National Science Foundation through grant number PHYS-1461251.  Any opinions, findings, and conclusions or recommendations expressed in this material are those of the author(s) and do not necessarily reflect the views of the National Science Foundation.

Project Overview: Studying nanoscale properties of molecular motion, energy, and geometry has developed hand in hand with laser optics. Evidence of this is best conveyed with the excitement over the “femtosecond pump-probe” experiments that are being performed here in the James R. Macdonald Laboratory at Kansas State University. Under the direction of Artem Rudenko, Daniel Rolles, and many others I studied the fundamentals of single pulse laser-molecule interactions with various important organic compounds. We observed fragmentation patterns and energies associated with such interactions.  Inevitably, the results we found from this single pulse procedure will be applied to more sophisticated pump-probe experiments. We will be able to perform these experiments with more efficiency and accuracy than ever before with the help from a newly equipped interferometric delay stage, which I helped build.

1. Background and Motivation:

A great deal of effort has been invested into the study of light induced isomerization of various organic structures. Understanding the molecular dynamics of such ultrafast and tortuous phenomenon will convey the underlying mechanisms for biological properties such as isomerization of receptor molecules in the eyes of animals or the photosystems of plants (1),(2). Because of this budding interest, optical and molecular physicists have experimented with the fairly simple organic molecule 1,3-cyclohexadiene . Over five decades have been spent studying this molecule, and nearly one hundred papers have been published with many enlightening results about this molecule. High time-resolve experiments have revealed the necessary conditions for the molecules signature rotational motion,and the extremely quick transition times to higher order molecular energy levels have been measured. The desire to study this molecule still pervades because higher order energy levels still remain unexplored and the carbon-ring nature of Cyclohexadiene acts as a stepping stone for more complicated organic molecules (3). Also, the ring-opening isomerization can play a crucial role in developing molecular switches for whole new levels of technology (4).

But you have to learn to walk before you can run. So, instead of implementing the mentioned high time-resolve methods of pump probe microscopy, we ionize the molecule using a single pulse 500 nm laser. A net absorption of one or two photons from the laser pulse can cause ionization to occur. After this ionization, what results is positive charge distributions throughout the geometry of the molecule. These newly spawned positive ions will of course repel each other in an ultrafast dynamic called Coulomb Explosion (5). For my overall goal, I wish to observe the types of resulting fragments and associated kinetic energy released after Cyclohexadiene goes through this Coulomb Explosion. There are various methods in order to achieve this goal, mostly involving time of flight mass –to-charge spectroscopy. Also we will use PIPICO and TRIPICO analysis, with which one can identify the two-body and three-body fragmentation, respectively, of the parent cyclohexadiene molecule after Coulomb Explosion. If all the fragmentation species can be identified, and the results adhere to the known parameters of the experiment, matching the previous results of pathway isomerization, I can consider this experiment a success.

2. Setup and Experimental Technique:

2.1 PULSAR

For ionization of molecules, high intense light sources are necessary. In order to probe or control intermolecular interactions, ultrafast light sources are necessary. Fortunately JRM labs is equipped with the state of the art PULSAR Laser for such purposes (Pulsar, standing for Prarie Ultrafast Light Source for Attosecond Research). For this experiment, we focused the laser beam onto a parabolic mirror in order to focus it to the accuracy of a few microns. This assures that laser-sample interaction has high probability. It also helps reduce the occurrence of multi-molecule ionization. We desire only a single molecule to interact with each laser pulse, in order to prevent false coincidences, where it appears that two fragments originate from the same parent molecule, when they actually don’t.

2.2 COLTRIMS

In order to observe fragmentation patterns after laser interaction, we utilized COLTRIMS techniques (COLTRIMS stands for COld Target Recoil Ion Momentum Spectroscopy). The COLTRIMS system begins with the ejection of a supersonic gas jet, which is so cold that the thermal momentum spread of the molecules is essentially negligible (5). The necessity of such cold temperatures is so that we can utilize the conservation of momentum, described later.

The COLTRIMS setup also contain an ultrahigh vacuum chamber, that uses a five stage differential pumping mechanism to assure that residual and unwanted particles are removed. After pumping, the chamber pressure is about Torr, or about atmospheric units. This is absolutely necessary; we only want detection 1-3 cyclohexadiene ionization, and data reading would be nearly impossible if a lot ambient molecules were also ionized.

Within the vacuum chamber, an adjustable electrostatic field is applied. The field is used to increase the kinetic energy of the ions, as well as guide them onto a detector at the end of the chamber. The electric field is generated by uniformly varying the voltage between the anode and the cathode detectors.

Ionization can be considered instantaneous, so at the moment of laser triggering, time values begin to be calculated. The moment of laser triggering is determined by a photodiode before entering the vacuum chamber. The time is calculated from the moment of laser incidence until the ions are detected on microchannel plate (MCP). The highly energized ion collides with the detector. Then a large chain reaction of highly energized electrons come flying off from the detector. This chain reaction makes it easy to determine the “stop-time” for the data acquisition.

At the back of the MCP is a delay line detector, which determines where the ion lands on the detector. The way it works is taking advantage of time differences between detection. The avalanche of electrons on the MCP will send out a signal in both directions to the edge of the detector. The propagation speed of this signal is constant in all directions. If the impact is off-center, the signal will be processed at different times, and the difference between these times has a direct relationship to the position on the detector.

Using detection time-difference to calculate the X-coordinate.

Using detection time-difference to calculate the Y-Coordinate.

All the values we wish to know from the light-matter interaction can be determined by momentum and energy of the fragments. In order to calculate such things, it is necessary to calibrate the time-of-flight and determine what fragmentation species result after coulomb explosion.

2.3 Calibration

In order to calibrate the time-of-flight detected, it helps to determine some theoretical values. This can be done easily by simply solving for the equation of motion of any ion. We begin by noting that the only force the ion experiences is that applied to the electric field .

Solving the quadratic equation for gives us a value for time-of-flight…

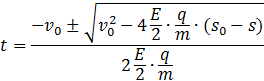
… and plugging in the appropriate parameters where we know that the initial position and velocity is zero…

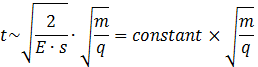
The above derivation makes the erroneous assumption of linear motion for the fragment in the chamber. To adjust for this, we add an offset time to the above time of flight expression, which is approximately the same for all ions. Now we can calibrate the time-of-flight spectrum, here we look at two high incident fragments and their distinctive time-of-flights, and solve for the two constants in the equations below.

As an example, for run 209, I saw a very distinct pattern for water and for the singly ionized parent cyclohexadine . Using these parameters I was able to solve for the unknown constants in the above equations.

Then, solving for the mass charge ratio , and inserting time-of-flight values of high incidence, we can see what kinds of molecules result from fragmentation.

Finally, with proper orientation of positioning on the detector and time-of-flights calibrated, we can solve for the initial momentum vectors of the fragments after coulomb explosion in all three directions.

Momentum in the z-direction.

Momentum in the x-direction.

Momentum in the y-direction.

Calculating the momentum of the fragments is important not only for the sake of knowing the momentum and energy of fragments from coulomb explosion, it also helps us distinguish good detection events versus bad ones. The “good events” are those that fulfill the conservation of momentum requirement, specifically those momentum vectors that sum to zero. This is justifiable for a few reasons; know the initial momentum of the parent molecule is zero (see discussion of gas jet above), and we know the momentum absorbed photons and emitted electrons will be negligible in comparison to the ions after acceleration in the chamber.

3 Data and Discussion:

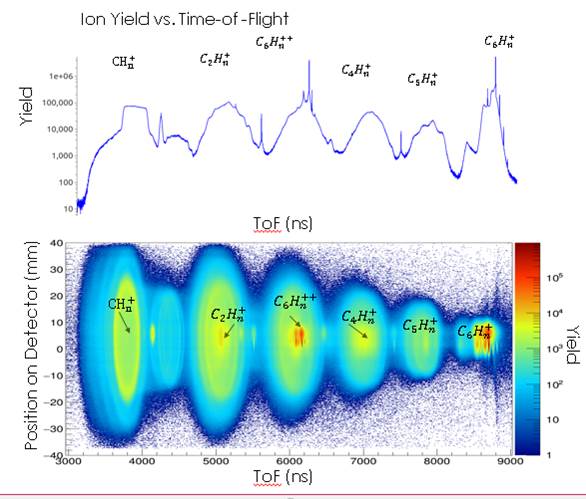
As the Time-of-Flight spectrums above show, a lot of incidence has occurred in this experiment. However, we are primarily interested in cases of the parent cyclohexadiene into two and three ionic fragments. Observing these cases and adhering to the COLTRIMS measurement techniques, we can look at released energy values, the geometry of breakup and compare them to the predicted isomerization that is discussed in the theory section of this report. The techniques of two-body fragmentation is called PIPICO. The techniques of three body breakup are called TriPICO. All these techniques are discussed here.

3.1 Two Body Breakup:

When the parent cyclohexadiene loses two electrons, the resulting charge distribution could be in such a way that two positively charged ions result. Because of their like charges, they will repel each other with the energy determined by the Coulomb potential, converting such energy into kinetic energy as they fly apart. This interaction is the aforementioned Coulomb explosion for two ions. Cases for this two-body breakup can be seen on a PhotoIon-PhotoIon-Coincidence (PIPICO) spectrum.

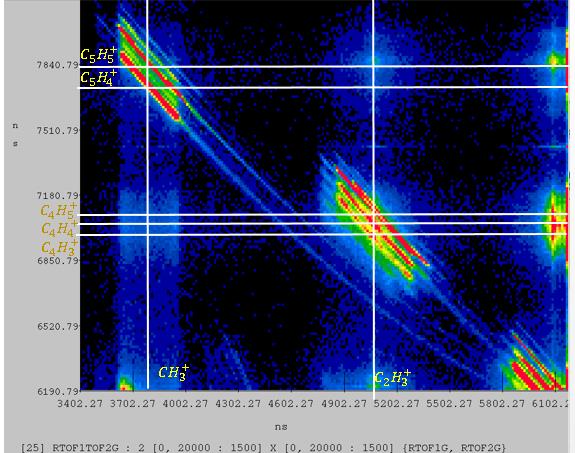
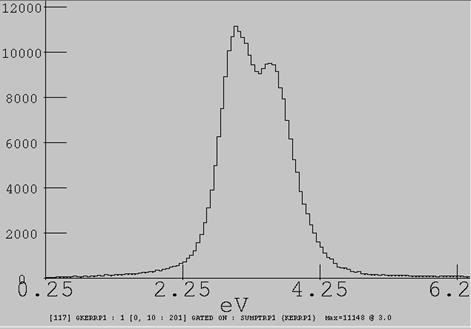
PIPICO spectrum shows cases where two species result from the same laser shot. The x-axis signifies the first ion that is detected, it will always have a smaller time-of-flight. Therefore it will always be the smaller fragment. The y-axis signifies the second ion that is detected, so it will always be the larger of the two fragments. Cases of high incidence with the linear fashion shown on the color gradient signify the two-body breakup that fulfills the conservation of momentum conditions.

The first annotated coordinate shows the events where the parent molecule is doubly ionized and fragments as . The highest yield for the kinetic energy released from this coulomb explosion centers around 3.00 eV to 3.20 eV, depending on the parameters of the laser and the applied electric field.

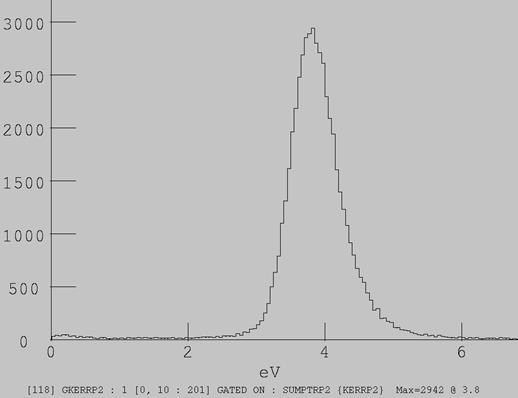
The second annotation shows the breakup. This has a slightly larger kinetic enrgy associated with it, centering around 3.80 eV.

3.2 Three body breakup:

In cases where the cyclohexadiene is triply ionized, a coulomb repulsion between three fragments will occur. These cases have much more interesting results because a lot of different geometries occur, even for the same ionic resultants. Also, the types of fragments reveal possible chain-opening isomerization that has been discovered in previous experiments, as discussed in the theory section.

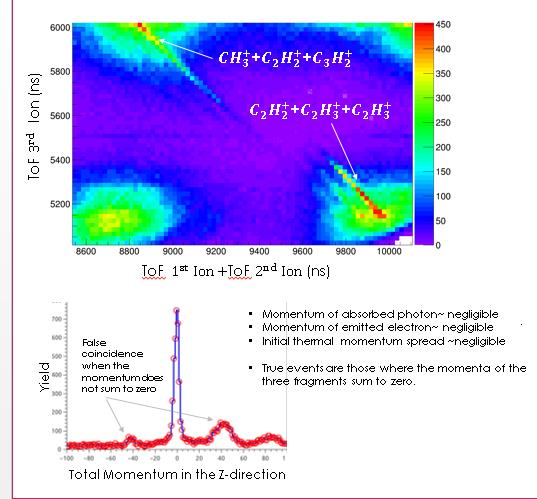
The TriPICO spectrum shows cases where three species result from a single laser incidence. The x-axis shows the time-of –flight for the first ion that is detected. The y-axis is the sum of the time-of-flights for the second and third ion that is detected. Once again, cases of high incidence with the linear fashion shown on the color gradient signify breakups that fulfill the conservation of momentum we implement.

The most apparent result of this TriPICO analysis is the symmetric breakup, . In cases of this symmetric breakup, we observe different distributions of the kinetic energy amongst the fragments, as well as different geometries of the breakup. In order to quantify this phenomenon 2D-rendered

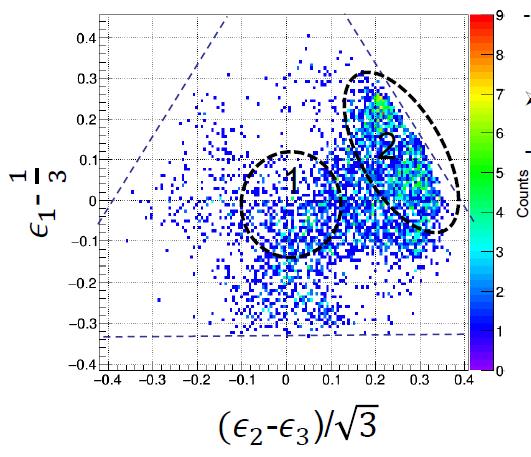
The above diagram is a Dalitz Plot. Using this plot allows us to read the energy distributions of three fragments on a single diagram. Areas of high incidence determined by the color gradient show which fragment received more kinetic energy after Coulomb explosion, depending on the events position on the inlaying circle. A brief description on how Dalitz Plots are formulated is in order:

0

0

G

F

E

D

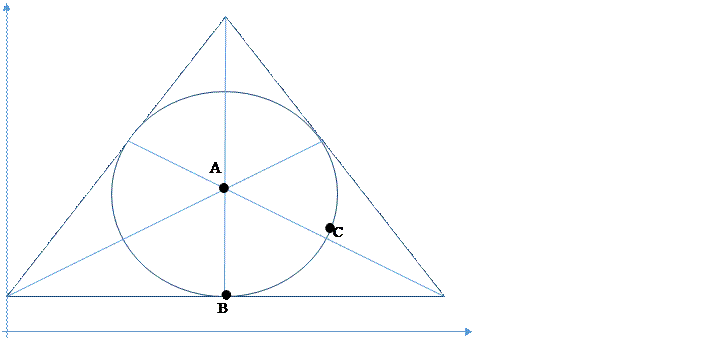
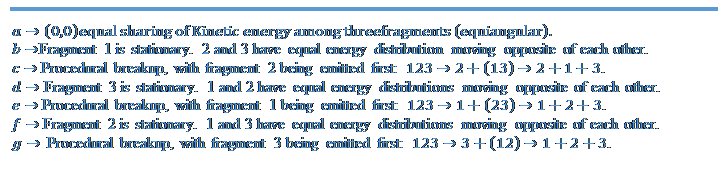
Dalitz Plots are defined in terms of a particles reduced energy. Reduced energy is a unit less value determined by proportionality of momentums.

Definition of reduced energy

All reduced energies of the fragments are normalized

· The axes of the Dalitz plots are defined by differences of the particles reduced energies.

Horizontal axis of a Dalitz plot

Vertical axis on a Dalitz plot

In our calibration procedure for symmetric breakup, we assigned fragment as and fragments 2 and 3 as.

The Dalitz Plot presenting the data above shows two cases of symmetric breakup.

1. This is the case of equal energy distribution and equiangular geometry. This is the scenario that is expected for coulomb explosion of a closed ring. Plotting the energy distribution for all three fragments, we see a value of about 3.8 eV. Also, the angular distribution of these fragments has a peak of the obvious .

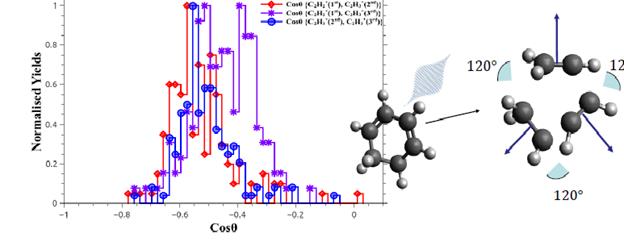
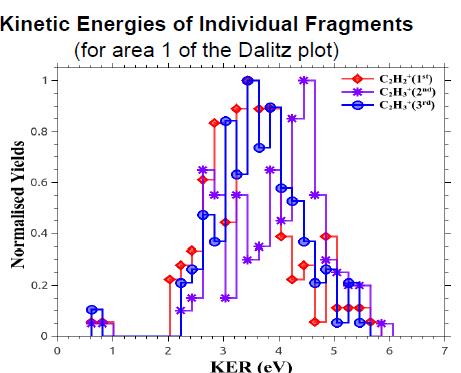
2. This scenario is the case of procedural breakup. Where a ion is left stationary. This case gives hint of possible ring opening of CHD to HT isomerization. This isomerization has been witnessed before, and suppression of this kind of isomerization can be done when a delayed laser pulse hits the molecule 50 fs after initial laser excitation [6].

4. Review and Conclusion

In this work, we used a 25 fs laser pulse in order to ionize the ring-structured molecule 1,3-cyclohexadiene. Frequent cases of multiple ionization occurred, which lead to two or three charged ions repelling each other. This repulsion lead to intense dynamics associated with the release of kinetic energy in a phenomenon called coulomb explosion.

We saw frequent cases of two body break up: channels of and . Also, we focused on the rich information obtained from symmetric three body breakup of . In this three-body coincidence, we see a lot of cases of equiangular geometry and equal kinetic energy among all three fragments. Other cases of unequal energy sharing give hints of a pathway in which the molecular geometry varies during ionization.

So now, we have a few fragmentation patterns that we would like to observe further. With the new delay stage attached and harmonic generator, we will be able to perform pump-probe laser microscopy. Using this technique, a single infrared pulse will induce excitation of the molecule to higher energy levels, and after a certain, controllable, delay time, a second x-ray will ionize it. Then we can perform these Coulomb Explosion techniques to determine the time-dependent dynamics of the molecule.

[1] Wang, Q., R. Schoenlein, L. Peteanu, R. Mathies, and C. Shank. "Vibrationally Coherent Photochemistry in the Femtosecond Primary Event of Vision." Science 2665184 (1994): 422-24.

[2] Zubik, Monika, Rafał Luchowski, Wojciech Grudzinski, Małgorzata Gospodarek, Ignacy Gryczynski, Zygmunt Gryczynski, Jerzy W. Dobrucki, and Wiesław I. Gruszecki. "Light-induced Isomerization of the LHCII-bound Xanthophyll Neoxanthin: Possible Implications for Photoprotection in Plants." Biochimica Et Biophysica Acta (BBA) - Bioenergetics 1807.9 (2011): 1237-243.

[3] Pullen S.H., Anderson N.A., Walker L.A., Sension R.J. “The ultrafast photochemical ring-opening reaction of the 1,3-cyclohexadiene in cyclohexane.” J. Chem. Phys. (1995) 108:556–63

[4] Geppert, D., L. Seyfarth, and R. De Vivie-Riedle. "Laser Control Schemes for Molecular Switches." Applied Physics B Appl. Phys. B 79.8 (2004): 987-92.

[5] Maharjan, Chakra Man. Momentum Imaging Studies of Electron and Ion Dynamics in a Strong Laser Field. PhD thesis. 2007

[6] Ergler, Th., A. A. Rudenko, B. Feuerstein, K. Zrost, C. D. Schröter, R. Moshammer, and J. Ullrich. "Fragmentation of Molecules Studied with Laser-induced Coulomb Explosion Imaging and Femtosecond Pump-probe Experiments." (2006): n. pag. Web.

Thanks: Thanks to professor Artem Rudenko and Daniel Rolles for all their direction in the research at every step of the way. And thanks to everyone in the group: Farzaneh, Balram, and Shashank. They were with me through all experimental procedurals. Without them I wouldn’t have even known how to upload data... Utuq, Seyyed, Xiang ( I miss you…), Yubaraj (wish I could have met you), and Jeff.

About Me:  I attend College University of Nebraska of Omaha. I study Physics with a minor in Mathematics, and German. I like to sit on couches and listen to the Grateful Dead a lot…